Optical properties of a vibrationally modulated solid state mott insulator

ABSTRACT Optical pulses at THz and mid-infrared frequencies tuned to specific vibrational resonances modulate the lattice along chosen normal mode coordinates. In this way, solids can be

switched between competing electronic phases and new states are created. Here, we use vibrational modulation to make electronic interactions (Hubbard-_U_) in Mott-insulator time dependent.

Mid-infrared optical pulses excite localized molecular vibrations in ET-F2TCNQ, a prototypical one-dimensional Mott-insulator. A broadband ultrafast probe interrogates the resulting optical

spectrum between THz and visible frequencies. A red-shifted charge-transfer resonance is observed, consistent with a time-averaged reduction of the electronic correlation strength _U_.

Secondly, a sideband manifold inside of the Mott-gap appears, resulting from a periodically modulated _U_. The response is compared to computations based on a quantum-modulated dynamic

Hubbard model. Heuristic fitting suggests asymmetric holon-doublon coupling to the molecules and that electron double-occupancies strongly squeeze the vibrational mode. SIMILAR CONTENT BEING

VIEWED BY OTHERS PHONON-DRESSED STATES IN AN ORGANIC MOTT INSULATOR Article Open access 30 March 2022 EXCITON-ASSISTED LOW-ENERGY MAGNETIC EXCITATIONS IN A PHOTOEXCITED MOTT INSULATOR ON A

SQUARE LATTICE Article Open access 06 March 2023 TERAHERTZ RADIATION BY QUANTUM INTERFERENCE OF EXCITONS IN A ONE-DIMENSIONAL MOTT INSULATOR Article Open access 13 October 2023 INTRODUCTION

A Mott insulator is a solid with fractionally filled electronic bands in which charge carriers do not conduct because of their mutual repulsion. The resulting properties1 are believed to be

captured by Hubbard models2,3, where only the screened Coulomb interaction, i.e. the on-site Hubbard-_U_, is retained. This approach has been widely applied to polymers, transition metal

oxides, fullerenes and cuprates4,5,6,7,8,9. Moreover, the Hubbard model can effectively describe optically excited Mott insulators where hot quasi-particles are injected across the gap. The

quantum dynamics following such “photo-doping” has been extensively studied in the solid state10,11,12,13. Non-equilibrium Hubbard physics is routinely explored in ‘artificial solids’

realized by ultra-cold atoms trapped in optical lattices14,15,16,17. However, a powerful feature of these systems is the possibility to accurately and dynamically control the strength of

interactions through the Feshbach resonances and the bandwidth via the lattice depth18. In contrast, the ability to control the parameters of ‘real’ materials is far more restricted. While

the bandwidth can be statically controlled across a limited parameter space, by using pressure19 or chemical substitutions, the Hubbard-_U_ cannot easily be modified since it is dependent on

the atomic physics of each site. A promising pathway to such control are optical pulses at THz and mid-infrared frequencies, which can be tuned to specific vibrational resonances and

modulate the lattice along a chosen normal mode coordinate. Since the photon energies are far below those of the correlation gap, this technique has already been used to switch solids

between competing electronic phases20,21 and to create new states not found at equilibrium22. Here, we investigate how vibrational modulation influences the electronic interactions in a Mott

insulator. Crucially, the leading-order effect of exciting these localised modes is to dynamically modulate the on-site electronic wavefunction, thereby inducing temporal oscillations and

modifying the time-averaged Hubbard-_U_ interaction strength. The response of a strongly correlated electronic system to such vibrational modulation is not well understood, yet the dynamical

states created have new properties and exhibit less dissipation than in the case of quasi-particles excitations, opening up the potential for optical devices with superior thermal

management. RESULTS We investigate at room-temperature an organic Mott insulator, _bis_(ethylendithyo)-tetrathiafulvalene-difluorotetracyano-quinodimethane (ET-F2TCNQ), which is a

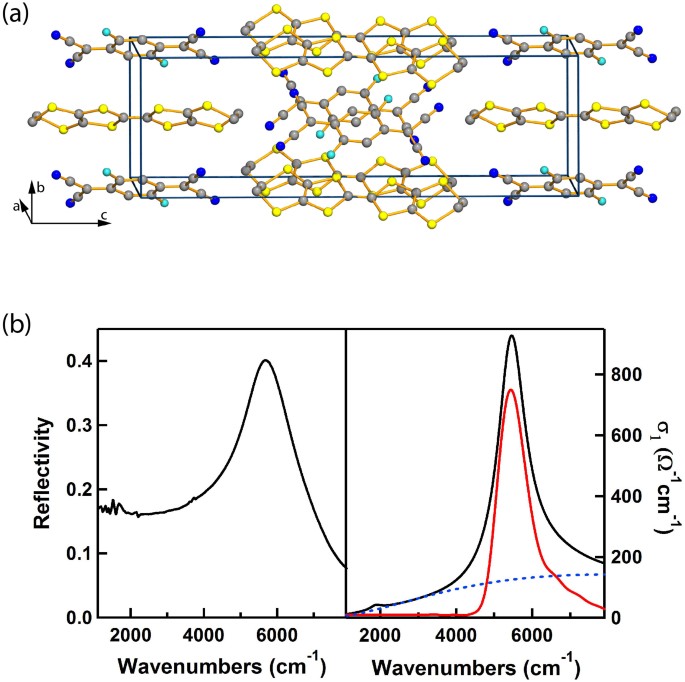
prototypical system where Hubbard physics accurately describes the electronic transport between individual molecular sites23. The static optical properties of ET-F2TCNQ are shown in figure 1

for light polarized along the chain of ET molecules. A charge-transfer resonance, corresponding to excitations of the type (ET+, ET+) → (ET2+, ET0) is observed at photon energies ~700 meV

(5500 cm−1), reflecting the existence of a correlation gap. In the time resolved experiments reported in this work, we measure the broadband optical properties as one localized

intramolecular mode is driven with mid-infrared femtosecond pulses. These were generated by difference-frequency mixing of two near-infrared pulses, obtained by parametric down-conversion of

a single 800 nm wavelength femtosecond pulse. The excitation pulses were polarized perpendicular to the chains, driving an infrared active molecular vibration close to ~10 μm wavelength (Ω

= 1000 cm−1)24. This 10 μm mode was excited with field strengths up to 10 MV/cm, thus strongly deforming the molecular oscillators and local molecular orbitals (see supplementary

information). The tuneable output of the second optical parametric amplifier was used to probe the reflectivity changes along the ET molecule chains in the mid (1800–3000 cm−1) and near

infrared (4000–7000 cm−1). The THz response was probed between 25 and 85 cm−1 using single-cycle pulses generated by optical rectification in ZnTe. When excited by the mid-infrared pump

pulses, a shift of the charge transfer band to lower frequency was observed, along with new distinct peaks inside the gap (figure 2a). In this driven state, no reflectivity change was

detected for frequencies above the charge transfer resonance and no metallic response was detected in the THz range, where the reflectivity remained low and the phonon resonances unscreened

(see figure 2b). The lack of a low frequency response clearly sets this modulated state apart from the photo-induced formation of metallic state by above gap optical excitation, for which a

Drude-like metallic response is typically observed25. Secondly, the rapid relaxation back to the ground state makes this experiment different from the case of the vibrationally driven phase

transitions, where THz frequency excitation can lead to switching between competing orders26,27. As the excited mode relaxes, the vibrational oscillations are reduced and the effects vanish

as seen in figure 2a. By 0.5 ps after the excitation, the peaks inside the gap are strongly reduced and the charge transfer band shifts back to its equilibrium position. Relaxation back to

the ground state occurred with a double-exponential decay (τ1 = 230 fs and τ2 = 4.5 ps). The first fast time constant we interpret as the direct relaxation of the system in the vibrational

excited state. The second long time constant we assign to a general thermalization of the hot mode with the whole crystal lattice that also involves low frequency phonon modes at THz

frequencies resulting in the ps-time constant. The response is also mode selective. When the excitation wavelength was tuned away from the frequency of this vibration, the mid-infrared

resonances disappeared. Quantitative analysis of the transient optical properties starts from fitting the broadband reflectivity spectra of figure 2b with a Drude-Lorentz Model and

transforming it into optical conductivity28. The conductivity lineouts are shown in figure 4c. The red-shift of the charge transfer band occurs from its equilibrium position at _ω/c_ ≈ 5500 

cm−1 toward 5000 cm−1 and a new band is observed approximately at 4200 cm−1. Additionally, a mid-gap resonance and a weaker peak appeared at ~3000 cm−1 and ~2000 cm−1, respectively. To

analyse these data, we start from a description of the static optical properties of this compound using the extended Hubbard Hamiltonian, In this expression and are creation and annihilation

operators for an electron at site with spin σ, is its corresponding number operator and . The optical response of the system at high photon energies is probed by the creation of Hubbard

excitons29 (figure 3a), composed of a doubly occupied “doublon” site with repulsive energy _U_, bound to an neighbouring empty “holon” site by a −_V_ attractive energy. The static optical

spectrum features a delta peak located at ω_CT_ = _U_ − _V_ − 4_t_2/_V_, corresponding to the Hubbard exciton and a broad continuum centered about _U_, with a bandwidth of _8t_,

corresponding to unbound holon-doublon excitations. Figure 1b shows that the measured spectrum is well fitted by the extended Hubbard model optical conductivity once a background

contribution from a high frequency oscillator (dashed blue curve) is added. To describe the vibrationally-driven state, we consider the model shown in figure 3b. To each molecular site we

explicitly introduce a harmonic oscillator describing the selected molecular mode that gives a _locally vibrating_ Hubbard model30 Here is the sum of local harmonic oscillator Hamiltonians

each with angular frequency Ω, displacement and ground state size α0. The term takes into account the coupling between the charge and driven mode and is expected to depend on the electronic

configuration as , where _f_ and _g_ are general functions. Typical electron-phonon interactions within the Holstein31 model would only retain a linear coupling to the charge density as .

However, a Holstein model is not sufficient to describe the “sloshing” motion of the infrared active distortion (see supplementary information) since by symmetry linear couplings vanish.

Instead we find (see Methods) that the vibration has a dipolar coupling to the charge sector which to leading order gives an interaction where and are the local holon and doublon projectors,

with respective positive couplings and . In addition to coupling to the vibration quadratically, crucially it also depends on the double occupancy as making a form of dynamic Hubbard

model27. This type of model, which is natural extension of the Holstein-Hubbard physics, was originally introduced by Hirsch27 to energetically account for reductions in the Hubbard _U_

arising from two electrons occupying the same site. As we shall show the similarity of this effect to the vibrational driving here necessitates the use of a dynamic Hubbard model in order to

capture the experimental findings. DISCUSSION To analyse the experimental data of figure 2 we computed the real part of the optical conductivity σ(ω) of via the unequal time current-current

correlation function32,33 χ_jj_(ω). The narrow hopping bandwidth of ET-F2TCNQ, as well as the strong binding of holon-doublon pairs, justifies σ(ω) being calculated analytically in the

_atomic__limit_ where electron hopping is neglected (see Methods). The key effect of vibrational excitation are revealed by considering the infinitely heavy oscillator limit, where its

classical displacement varies harmonically in time τ as _q_ = _Q_cos(Ωτ), with amplitude _Q_ and Ω = 1000 cm−1 the frequency of the driven molecular vibration. Thus, the onsite interaction

matrix element _U_ is modulated by (_h_ − _d_)_q_2 and results in two crucial features. First, the _q_2 ∝ _Q_2[1 + cos(2Ωτ)] dependence predicts a shift of the charge transfer resonance, by

an amount that depends on the amplitude of the driven mode _Q_. The charge transfer resonance is expected to red-shift if the doublon coupling exceeds that of the holon and therefore the

time-averaged _U_ is effectively reduced. A blue-shift occurs for the opposite case. Second, the classical frequency modulation generates sidebands at multiples of ±2 Ω on each side of the

shifted charge transfer resonance. That a shift in the charge transfer resonance is seen in figure 2a, along with the emergence of a mid-gap peak at 2 Ω below, is strong evidence of the

quadratic modulation of the interaction _U_ consistent with the dynamic Hubbard model. A number of experimental features are not captured by the simple classical modulation picture. In

particular a sideband is only seen to the red of the charge transfer resonance and additional sub-structure is present. Instead, to better reproduce these experimental line shapes with a

dynamic Hubbard model implies a strong holon-doublon asymmetry along with a quantum treatment of a finite mass oscillator (see Methods). As shown in figure 3b, this strongly coupled limit

results in back-action of the electronic configuration on the vibrational mode. Specifically, describes a “stiffening” of the oscillator on the holon site, so Ω_h_ > Ω and a “slackening”

on the doublon site, so Ω_d_ < Ω34,35. Such abrupt frequency changes are well known to cause squeezing of the oscillators dynamics36,37. Figure 4b and 4c show the comparison with the

optical conductivity extracted from the reflectivity data and a calculation of σ(ω) with strong coupling aimed to reproduce the overall structure of the experimental conductivity data. We

obtain a driving strength _Q_/α0 ≈ 2, a doublon oscillator that suffers a significant frequency reduction to Ω_d_ ≈ 0.26 Ω and a holon's Ω_h_ ≈ 1.10 Ω that is only marginally increased.

Therefore the additional sub-structure seen in figure 4b can be attributed to the “slackening” of the doublon oscillator as schematically shown in figure 4a. Crucially, the reduced spacing

between energy levels of the doublon oscillator causes the transition frequencies to move into the gap, an effect that is significant only if the vibrational mode is appreciably populated as

found from the model parameters to fit the data. This finding is in agreement with the strong experimental excitation of the molecular oscillator. The remnants of the classical sidebands

are now located only at low frequencies and are split into multiples of Ω − Ω_d_. We note that the 10% modulation of the holon frequency is in agreement with the expectations34,35 for a

thermal charge ordered molecular state. However, the substantial reduction, by a factor 4, of the frequency for the doublon site goes far beyond any equilibrium predictions. Nonetheless, the

greater effect is consistent with the charge distribution of double occupancies lying predominantly near the oscillating C = C bond38,39. By way of comparison, we also report the response

when tuning the pump wavelength to 6 μm, near a weakly infrared-active symmetric mode along the chains40. This vibrational mode is expected to have a linear coupling. As shown in figure 5,

only a reduction in spectral weight at the charge transfer resonance was observed, without any shift or significant response at other wavelengths. As expected, this indicates a dependence of

the optical response on the coupling strength, driving and symmetry of the selected vibrational mode. This experiment demonstrates how for this compound selective vibrational excitation of

intramolecular modes can, to first approximation, cause a dynamical modulation of the on-site Hubbard-_U_ interaction. Such control is not easily accomplished by other means and the method

is likely to be applicable to a broader class of organic materials. More refined analysis of the observed lineshape carries important information about the microscopic interactions of the

material, such as holon-doublon asymmetry. Most importantly, the response to such strongly driven vibrational modes reveals the strength of their coupling to the electronic structure. More

generally, the selective modulation of one degree of freedom, when combined with probing of the electronic spectrum, raises the tantalising prospect of experimentally _deconstructing_ the

Hubbard Hamiltonian, by exposing one specific coupling that would otherwise have a vanishingly small contribution to the equilibrium properties. The deconstruction obtained by such quantum

modulation spectroscopy should be applicable to more than one class of modes, including charge, magnetic or orbital excitations. METHODS COUPLING OF LOCAL MODES TO ELECTRONS In general a

spin-independent coupling between a local harmonic oscillator and the electronic configuration of a site can be written in terms of and only, as the number operator is fermionic. We assume

that this coupling can be expressed as , where and are two functions of the local mode coordinate that are not known a priori. By expanding the functions _f_ and _g_ into a Taylor series we

obtain with coupling constants _A__i_, _B__i_ which are constrained by the symmetry of the molecular modes41. An antisymmetric infrared vibration, such as the 10-μm mode in the experiment,

is akin to an oscillating dipole. Within the Born-Oppenheimer approximation this perturbation causes admixing of the valence orbital with higher-lying excited states of differing parity and

induces an energy shift that is an even function of , meaning e.g. that _A_1 = 0. This already precludes that such a vibration can be described by a conventional Holstein-Hubbard31 type

interaction. In contrast, a symmetric Raman vibration, such as the mode at 6 μm, is instead captured by an oscillating quadrupole, admixing higher-lying states of the same parity and causing

a linear energy shift with non-zero _A_1. The second term in the expression for , which includes coupling to the double occupancy, is determined by computing the Coulomb repulsion arising

from both electrons occupying the admixed vibrational orbital via where is the distance between the electrons. Retaining these terms is crucial to properly describing how vibrations modify

the on-site Hubbard _U_ electronic interactions. For the infrared vibration the differing parity of the states in the admixture causes _U_(_q_) to again vary, to lowest order, quadratically

with displacement _q_ (implying _B_1 = 0), while the Raman vibration retains a linear dependence. It is found that _B_2 < 0 for the infrared mode because the admixed vibrational orbital

spatially expands for any non-zero displacement in this simple model. We finally re-arrange terms to isolate the holon and doublon couplings and to give the expression for in the main text.

OPTICAL CONDUCTIVITY OF A VIBRATING HUBBARD CHAIN Since the system does not display any long-range Neel spin-order and does not contain doublons in its thermal state. It is thus well

approximated by a half-filled completely spin-mixed state where is a spin configuration state of the electronic Mott insulator. Given that higher vibrational states of the localized

molecular oscillators are essentially unoccupied at room temperature. To model the vibrationally driven state we assume that each oscillator is prepared instantaneously in a coherent state .

The phase of the oscillator on each site is expected to be identical across the sample, however it is not controlled shot-to-shot in the experiment. As such a global averaging of the phase

of α is made. We further assume that the relative phase of the oscillators where the holon and doublon becomes incoherent upon optical excitation. Since in the atomic limit these are the

only relevant oscillators in the problem, this is equivalent to assuming they are both in a phase-averaged coherent state . To compute the optical conductivity we start from the unequal time

current-current correlation function which is defined as where Θ(τ) is the Heaviside function and is the Heisenberg picture current operator. The regular finite frequency optical

conductivity then follows as where χ_jj_(ω) and are the Fourier transforms of χ_jj_(τ) and its complex conjugate, respectively. In the atomic limit we obtain the current-current correlation

function29 as a convolution of the Hubbard shift and the onsite vibrational doublon and holon correlation functions where is the squeezing operator with and _p__n_ are the diagonal elements

of . The matrix elements weighting the δ functions are Franck-Condon factors and are well known analytically42,43. A similar analysis can proceed for the Raman mode. ATOMIC LIMIT AND HOPPING

The optical conductivity is calculated here in the so-called zero-bandwidth atomic limit, where the ratio σ1(ω)/_t_2 can be obtained exactly. This approach is justified by the parameters of

ET-F2TCNQ, which indicate that an adjacent holon-doublon pair will be bound. Thus, once optically excited the contributions of the oscillators at the initial holon and doublon locations

will be dominant. Moreover, in the dynamic Hubbard model , the quadratic electron-oscillator coupling causes a suppression of _t_, analogous to polaronic effects28. A further suppression of

the coherent hopping processes occurs once the vibrational modes are driven, similar to the reduction with temperature seen for polarons. For the strong coupling infrared case we estimate

that the hopping of holons drops to around 0.2 _t_, while for doublons it is just 0.05 _t._ Also, because the oscillator coupling here is to a _local_ molecular mode on each site, as opposed

to a _collective_ bath of lattice phonons, vibrational excitation disorders hopping through the chain, further inhibiting holon-doublon motion. Thus, the effects of a finite _t_ are to

broaden the dominant contribution to the optical conductivity already captured by the on-site vibrational dynamics. To account for these mechanisms, as well as the spectral limitations of

the measurement itself, we introduced an artificial broadening of 0.5 _t_ to the results presented. REFERENCES * Lee, P. A., Nagaosa, N. & Wen, X.-G. Doping a Mott insulator: Physics of

high-temperature superconductivity. Rev. Mod. Phys. 78, 17–85 (2006). Article  CAS  ADS  Google Scholar  * Mott, N. F. The Basis of the Electron Theory of Metals, with Special Reference to

the Transition Metals. Proc. Phys. Soc. (London) A62, 416–422 (1949). Article  ADS  Google Scholar  * Hubbard, J. Electron Correlations in Narrow Energy Bands. Proc. Roy. Soc. A 276, 238–257

(1963). Article  ADS  Google Scholar  * Hubbard, J. Generalized Wigner lattices in one dimension and some applications to tetracyanoquinodimethane (TCNQ) salts. Phys. Rev. B 17, 494–505

(1978). Article  CAS  ADS  Google Scholar  * Lee, W.-C. & Phillips, P. W. Spectral weight transfer in multiorbital Mott systems. Phys. Rev. B 84, 115101 (2011). Article  ADS  Google

Scholar  * Gunnarsson, O., Koch, E. & Martin, R. M. Mott transition in degenerate Hubbard models: Application to doped fullerenes. Phys. Rev. B 54, R11026–R11029 (1996). Article  CAS 

ADS  Google Scholar  * Wagner, J., Hanke, W. & Scalapino, D. J. Optical, magnetic and single-particle excitations in the multiband Hubbard model for cuprate superconductors. Phys Rev. B

43, 10517–10529 (1991). Article  CAS  ADS  Google Scholar  * The Hubbard Model: Its Physics and Mathematical Physics (Nato Science Series B). [Baeriswyl, D., Campbell, D. K., Carmelo, J. MP.

& Guinea, F. (ed.)] Springer, New York and London (1995). * Quintanilla, J. & Hooley, C. The strong-correlations puzzle. Physics World (June 2009), [32–37]. * Cavalleri, A. et al.

Femtosecond Structural Dynamics in VO2 during an Ultrafast Solid-Solid Phase Transition. Phys. Rev. Lett. 87, 237401 (2001). Article  CAS  ADS  Google Scholar  * Iwai, S. et al. Ultrafast

Optical Switching to a Metallic State by Photoinduced Mott Transition in a Halogen-Bridged Nickel-Chain Compound. Phys Rev. Lett. 91, 057401 (2003). Article  CAS  ADS  Google Scholar  *

Wall, S. et al. Quantum interference between charge excitation paths in a solid state Mott insulator. Nature Physics 7, 114–118 (2011). Article  CAS  ADS  Google Scholar  * Perfetti, L. et

al. Time Evolution of the Electronic Structure of 1T-TaS2 through the Insulator-Metal Transition. Phys. Rev. Lett. 97, 067402 (2006). Article  CAS  ADS  Google Scholar  * Greif, D. et al.

Short-Range Quantum Magnetism of Ultracold Fermions in an Optical Lattice. Science 340, 1307–1310 (2013). Article  CAS  ADS  Google Scholar  * Chen, Y.-A. et al. Controlling Correlated

Tunneling and Superexchange Interactions with ac-Driven Optical Lattices. Phys. Rev. Lett. 107, 210405 (2011). Article  ADS  Google Scholar  * Uehlinger, T. et al. Artificial graphene with

tunable interactions. _arXiv_:1308.4401 (2013). * Heinze, J. et al. Intrinsic Photoconductivity of Ultracold Fermions in Optical Lattices. Phys. Rev. Lett. 110, 085302 (2013). Article  CAS 

ADS  Google Scholar  * Bloch, I., Dalibard, J. & Zwerger, W. Many-body physics with ultracold gases. Rev. Mod. Phys. 80, 885–964 (2008). Article  CAS  ADS  Google Scholar  * Mitrano, M.

et al. Pressure tuning of ultrafast quasiparticle relaxation in a Mott insulator. _arXiv_:1308.2189 (2013). * Rini, M. et al. Control of the electronic phase of a manganite by mode-selective

vibrational excitation. Nature 449, 72–74 (2007). Article  CAS  ADS  Google Scholar  * Fausti, D. et al. Light induced Superconductivity in a Stripe-ordered Cuprate. Science 331, 189–191

(2011). Article  CAS  ADS  Google Scholar  * Kaiser, S. et al. Light-induced inhomogeneous superconductivity far above Tc in YBa2Cu3O6 + x . _arXiv_:1205.4661v5 (2013). * Hasegawa, T. et al.

Electronic states and anti-ferromagnetic order in mixed-stack charge-transfer compound (BEDT-TTF)(F2TCNQ). Solid State Comm. 103, 489–493 (1997). Article  CAS  ADS  Google Scholar  *

Kozlov, M. E., Pokhodniaa, K. I. & Yurchenkoa, A. A. The assignment of fundamental vibrations of BEDT-TTF and BEDT-TTF-d8 . Spectrochimica Acta Part A: Molecular Spectroscopy 43, 323–329

(1987). Article  ADS  Google Scholar  * Okamoto, H. et al. Photoinduced Metallic State Mediated by Spin-Charge Separation in a One-Dimensional Organic Mott Insulator. Phys Rev. Lett. 98,

037401 (2007). Article  CAS  ADS  Google Scholar  * Tobey, R. I. et al. Ultrafast Electronic Phase Transition in La1/2Sr3/2MnO4 by Coherent Vibrational Excitation: Evidence for Nonthermal

Melting of Orbital Order. Phys Rev. Lett. 101, 197404 (2008). Article  CAS  ADS  Google Scholar  * Caviglia, A. et al. Ultrafast Strain Engineering in Complex Oxide Heterostructures. Phys.

Rev. Lett. 108, 136801 (2012). Article  CAS  ADS  Google Scholar  * Dean, N. et al. Polaronic Conductivity in the Photoinduced Phase of 1T-TaS2 . Phys. Rev. Lett. 106, 016401 (2011). Article

  CAS  ADS  Google Scholar  * Essler, F. H. L., Gebhard, F. & Jeckelmann, E. Excitons in one-dimensional Mott insulators. Phys. Rev. B 64, 125119 (2001). Article  ADS  Google Scholar  *

Hirsch, J. E. Dynamic Hubbard Model. Phys. Rev. Lett. 87, 206402 (2001). Article  CAS  ADS  Google Scholar  * Holstein, T. Studies of polaron motion: Part I. The molecular-crystal model.

Ann. Phys. 8, 325–342 (1959). Article  CAS  ADS  Google Scholar  * Mahan, G. D. Many-Particle Physics 3rd ed. Plenum Pub. Corp.(2000). * Essler, F. H. L. et al. The One-Dimensional Hubbard

Model. Cambridge University Press (2005). * Yamamoto, T. et al. Examination of the Charge-Sensitive Vibrational Modes in Bis(ethylenedithio)tetrathiafulvalene. J. Phys. Chem. B 109,

15226–15235 (2005). Article  CAS  Google Scholar  * Girlando, A. Charge Sensitive Vibrations and Electron-Molecular Vibration Coupling in Bis(ethylenedithio)-tetrathiafulvalene (BEDT-TTF).

J. Phys. Chem. C 115, 19371–19378 (2011). Article  CAS  Google Scholar  * Agarwal, G. S. & Arun Kumar, S. Exact quantum-statistical dynamics of an oscillator with time-dependent

frequency and generation of nonclassical states. Phys. Rev. Lett. 67, 3665–3668 (1991). Article  CAS  ADS  Google Scholar  * Graham, R. Squeezing and Frequency Changes in Harmonic

Oscillations. J. Mod. Opt. 34, 873–879 (1987). Article  CAS  ADS  MathSciNet  Google Scholar  * Guionneau, P. et al. Determining the charge distribution in BEDT-TTF salts. Synthetic Metals

86, 1973–1974 (1997). Article  CAS  Google Scholar  * Imamura, Y. et al. Structures and electronic phases of the bis(ethylenedithio)tetrathiafulvalene (BEDT-TTF) clusters and κ-(BEDT-TTF)

salts: A theoretical study based on ab initio molecular orbital methods. J. Chem. Phys. 111, 5986–5994 (1999). Article  CAS  ADS  Google Scholar  * Rice, M. J. Towards the experimental

determination of the fundamental microscopic parameters of organic ion-radical compounds. Solid State Comm. 31, 93–98 (1979). Article  CAS  ADS  Google Scholar  * Sponer, H. & Teller, E.

Electronic Spectra of Polyatomic Molecules. Rev. Mod. Phys. 13, 75–170 (1941). Article  CAS  ADS  Google Scholar  * Kim, M. S. et al. Properties of squeezed number states and squeezed

thermal states. Phys. Rev. A 40, 2494–2503 (1989). Article  CAS  ADS  Google Scholar  * de Oliveira, F. A. M. et al. Properties of displaced number states. Phys. Rev. A 41, 2645–2652 (1990).

Article  CAS  ADS  Google Scholar  Download references AUTHOR INFORMATION Author notes * Kaiser S., Clark S. R. and Nicoletti D. contributed equally to this work. AUTHORS AND AFFILIATIONS *

Max-Planck-Institute for the Structure and Dynamics of Matter, Hamburg, Germany S. Kaiser, D. Nicoletti, G. Cotugno & A. Cavalleri * Centre for Quantum Technologies, National University

of Singapore, Singapore S. R. Clark & D. Jaksch * Department of Physics, Oxford University, Clarendon Laboratory, Parks Road, Oxford, UK S. R. Clark, G. Cotugno, R. I. Tobey, N. Dean, 

D. Jaksch & A. Cavalleri * CNR-IOM and Department of Physics, University of Rome “La Sapienza”, Rome, Italy S. Lupi * National Institute of Advanced Industrial Science and Technology,

Tsukuba, Japan T. Hasegawa * Department of Advanced Materials Science, University of Tokyo, Chiba, 277-8561, Japan H. Okamoto Authors * S. Kaiser View author publications You can also search

for this author inPubMed Google Scholar * S. R. Clark View author publications You can also search for this author inPubMed Google Scholar * D. Nicoletti View author publications You can

also search for this author inPubMed Google Scholar * G. Cotugno View author publications You can also search for this author inPubMed Google Scholar * R. I. Tobey View author publications

You can also search for this author inPubMed Google Scholar * N. Dean View author publications You can also search for this author inPubMed Google Scholar * S. Lupi View author publications

You can also search for this author inPubMed Google Scholar * H. Okamoto View author publications You can also search for this author inPubMed Google Scholar * T. Hasegawa View author

publications You can also search for this author inPubMed Google Scholar * D. Jaksch View author publications You can also search for this author inPubMed Google Scholar * A. Cavalleri View

author publications You can also search for this author inPubMed Google Scholar CONTRIBUTIONS S.K. and A.C. designed the research. S.K., D.N. and R.I.T. build the experimental apparatus and

performed measurements together with N.D. and S.L.; S.R.C., G.C. and D.J. developed the theoretical model and carried out the calculations presented. H.O. and T.H. provided and characterized

the samples. All the co-authors contributed to the analysis and discussion for the results. S.K., S.R.C. and A.C. wrote the paper and all the co-authors comment on it. ETHICS DECLARATIONS

COMPETING INTERESTS The authors declare no competing financial interests. ELECTRONIC SUPPLEMENTARY MATERIAL SUPPLEMENTARY INFORMATION Supplementary information: Optical Properties of a

Vibrationally Modulated Solid State Mott Insulator RIGHTS AND PERMISSIONS This work is licensed under a Creative Commons Attribution-NonCommercial-NoDerivs 3.0 Unported License. To view a

copy of this license, visit http://creativecommons.org/licenses/by-nc-nd/3.0/ Reprints and permissions ABOUT THIS ARTICLE CITE THIS ARTICLE Kaiser, S., Clark, S., Nicoletti, D. _et al._

Optical Properties of a Vibrationally Modulated Solid State Mott Insulator. _Sci Rep_ 4, 3823 (2014). https://doi.org/10.1038/srep03823 Download citation * Received: 08 October 2013 *

Accepted: 03 January 2014 * Published: 22 January 2014 * DOI: https://doi.org/10.1038/srep03823 SHARE THIS ARTICLE Anyone you share the following link with will be able to read this content:

Get shareable link Sorry, a shareable link is not currently available for this article. Copy to clipboard Provided by the Springer Nature SharedIt content-sharing initiative