A mass-spring model unveils the morphogenesis of phototrophic diatoma biofilms

ABSTRACT Diatoms often dominate planktonic communities in the ocean and phototrophic biofilms in streams and rivers, greatly contributing to global biogeochemical fluxes. In pelagic

ecosystems, these microscopic algae can form chain-like microcolonies, which seem advantageous for nutrient uptake and protect against grazing and at the same time reduce sinking. Despite

the capability of many diatoms to form chains, their contribution to the architecture of phototrophic biofilms remains elusive. Here we propose a computational model to simulate the growth

and behaviour of _Diatoma_ chains in contrasting flow environments. This mass-spring mechanical model captures the natural behaviour of _Diatoma_ chains well, emphasising the relevance of

chain growth and entanglement for biofilm morphogenesis. The model qualitatively describes formation of intricate dome-shaped structures and of dreadlock-type streamers as observed in nature

in multidirectional and unidirectional flow, respectively. The proposed model is a useful tool to study the effect of fluid dynamics on biofilm morphogenesis. SIMILAR CONTENT BEING VIEWED

BY OTHERS MODULATION OF BIOFILM GROWTH BY SHEAR AND FLUCTUATIONS IN TURBULENT ENVIRONMENTS Article Open access 11 April 2025 A LAGRANGIAN MODEL FOR DRIFTING ECOSYSTEMS REVEALS

HETEROGENEITY-DRIVEN ENHANCEMENT OF MARINE PLANKTON BLOOMS Article Open access 29 September 2023 EXTREME FLOW SIMULATIONS REVEAL SKELETAL ADAPTATIONS OF DEEP-SEA SPONGES Article 21 July 2021

INTRODUCTION Diatoms are the most successful microscopic algae and greatly contribute to the global biogeochemical machinery1. In the ocean, small-scale turbulence that affects the

replenishment of nutrients2 and constrains microcolony formation3,4 is key to the ecology and evolution of planktonic diatoms. In streams and rivers, diatoms are essential building-blocks of

benthic phototrophic biofilms — surface-attached and matrix-enclosed microbial communities — and are relevant, for instance, for primary production and sediment stabilization5,6. Overall,

benthic biofilms dominate microbial life in streams and rivers, where they control vital ecosystem functions and even influence large-scale carbon fluxes7,8. As a seemingly universal

feature, biofilms can form, depending on the fine-scale hydrodynamics, conspicuous physical structures such as filamentous streamers that oscillate in the flow9,10 or star-like features11.

In monospecies bacterial biofilms, the morphogenesis of such structures depends only on the constituent bacterial cells and their extracellular polymeric substances (EPS)12. In streams and

rivers, however, biofilms are not monospecies communities; they include prokaryotes, microalgae, small metazoans and various organic and inorganic particles and their organisation is

therefore very complex. Yet unresolved questions remain: how can apparently universal structures emerge in the phototrophic biofilms governing benthic life and what is the contribution of

diatoms to the architecture of these biofilms? In this study, we used empirical observations on the formation of phototrophic biofilms dominated by chain-forming diatoms and created a model

of diatom chain growth and biofilm morphogenesis in different hydrodynamic regimes. Biofilms were grown in streamside flumes under contrasting flow conditions reflecting the full complexity

occurring in low-emergence streams. The growth and behaviour of the _Diatoma_ chains were modelled assuming a system of particles connected by springs as is often done to model flexible

structures. This approach has proven successful to model, for example, the behaviour of hair strips13, protein folding14 and realistic rendering of cloth15. Mass-spring models have also been

used to explain patterns which develop during the social movement of myxobacteria16,17. Here, populations consisting of thousands of flexible rod-shaped cells gliding on a substratum

propelled by a motility engine were shown to form specific patterns, function of the diverse forces acting on each bacterial body and multiple cell-cell interactions. A similar approach was

used to represent trichomes of gliding cyanobacteria and to quantify the effectiveness of photophobic responses in large populations of long flexible filaments18. For biofilm modelling,

several mass-spring models have also been reported. A mass-spring model was used to study cell attachment in flow channels19 and a coupled particle-spring mechanical model with immersed

boundary method was built to study biofilm deformation under various flow conditions20. In this study, a mass-spring model of flexible _Diatoma_ chains was exposed to processes of movement

due to flow, cell growth, chain collisions, sticking and cell attachment. The model is a useful theoretical tool to study the interaction between fluid flow and biofilm morphogenesis. Our

study explains how under slow and multidirectional flow, intricate dome-shaped _Diatoma_ structures are formed, whereas elongated, dreadlock-type filamentous structures develop in fast and

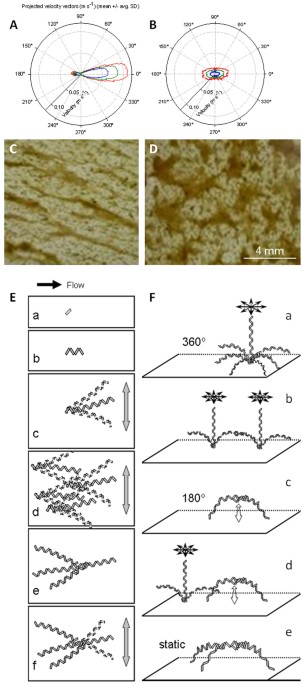
mostly unidirectional flow. RESULTS EMPIRICAL OBSERVATIONS Triangular bedforms (8 cm high) were used to induce varying hydrodynamic conditions in experimental flumes11,21. At the crest of

these bedforms, the flow was largely unidirectional with an average speed of 0.13±0.01 m s−1 (Figure 1A). In the trough between consecutive bedforms, eddies imposed multidirectionality on

the flow, including variation in the vertical dimension. The flow velocity averaged 0.04±0.01 m s−1 (Figure 1B). In both microenvironments, _Diatoma_ cells (length: ca. 50 μm; width: ca. 5 

μm) dominated nascent phototrophic biofilms and developed chains up to several millimetres in length. However, these chains ultimately developed differing architectures in the contrasting

hydrodynamic microenvironments. Ripple-like structures and streamers characterised biofilms at the crest (Figure 1C,E) whereas Y-shaped structures were abundant in the biofilms growing in

the trough (Figure 1D,F). We observed that in multidirectional flow, individual _Diatoma_ chains can rotate 360° around their base (Figure 1Fa) thus increasing the probability to encounter

neighbouring chains (Figure 1Fb). The entanglement of two neighbouring _Diatoma_ chains produces an arch which is restricted to a 180° flapping movement in the water (Figure 1Fc). This arch

can further grow in height due to cell division but can also expand in width due to particle sequestration from streaming water. Next, if an arch becomes further entangled with a

neighbouring _Diatoma_ chain that rotates in the flow, a Y-shaped structure emerges forming the backbone of the observed irregular quasi-polygonal structures (Figure 1Fd). In contrast,

unidirectional flow at the crest constrains the movement of _Diatoma_ chains forcing them to entangle into elongated streamers that still float above the substratum (Figure 1E). MODEL

PROCESSES To explain chain formation and behaviour of _Diatoma_ cells in contrasting flow environments, a mass-spring numerical model, summarized in the _Methods_ section and detailed in the

_Supplementary Methods_ online, has been developed. In the model, individual _Diatoma_ cells are connected by an EPS pad, which results in a zigzagging chain (Figure 2A,B). Such a chain,

made up of _n_ cells, is modelled as an array of _n_+1 particles with mass, which are connected by three springs of different type (Figure 2C). First-order springs connect adjacent particles

to construct the body of each _Diatoma_ cell. These springs are stiff because the silicate cell wall of _Diatoma_ cells does not allow for elongation or deformation. Second-order springs

connect every second particle to form the zigzagging shape of the chain. These springs are more flexible than first-order springs because the intercellular EPS pad allows for angles within a

measured range of 115.7° to 128.2° between two cells. Third-order springs connect every third particle in the chain to prevent rotation and deformation of the generated zigzags. The

movement of individual _Diatoma_ cells was modelled taking into account not only drag and lift forces from the fluid flow, elastic forces of the _Diatoma_ cell and collision forces between

_Diatoma_ chains, but also chain growth and chain-substratum attachment phenomena in a three-dimensional Cartesian coordinate system reflecting flow environment conditions measured _in situ_

as described in the _Methods_ section. Studies exist to determine the mechanical properties of zigzagged diatom chains4. Measurement of the flexural stiffness of the _Diatoma_ cells and of

the intercellular EPS bonds is not likely to provide accurate constants for all three springs required for the simulations. Spring constants were thus chosen empirically by performing

sensitivity analyses (_Supplementary Table S1_ and _Figure S1_). The constants for the wall-, collision- and sticking-springs were set ten-fold weaker than the constitutive springs to allow

for flexibility in the contacts. Setting the springs too stiff resulted in chains of cells that did not respond to hydrodynamics, while setting the springs too weak resulted in “escape” of

the chains, unrealistic stretching and loss of the characteristic zigzagging pattern. A moderate value was therefore retained, such that filaments resembled realistic microcolonies.

Simulations showed that once _Diatoma_ chains oscillating in the flow collide, they irreversibly connect and thereby increase the chance of further collisions with neighbouring chains. This

leads to the formation of complicated structures. The probability to encounter and to stick together was set low enough in the model to ensure that this process did not visibly overwhelm the

system with massive knotting of the _Diatoma_ chains, but it was high enough to allow “dreadlock” formation, for instance. Sticking leads not only to intertwining of chains, but indeed also

to the formation of arches as observed in natural biofilms. Figure 3 (a–c) shows the formation of such an arch upon sticking of a long chain to a short attached chain. When embedded in the

hydrodynamic microenvironment characteristic for the crest and the trough, respectively, the proposed model provided a reasonably realistic picture of the _Diatoma_ chain architecture and

behaviour (Figure 4 and _Supplementary Videos SV1 and SV2_ ). In the trough, zigzagged _Diatoma_ chains developed rapidly over two days and moved following the flow patterns of water. After

a lag phase of growth and interactions, chains of cells that moved in the water flow started to become entangled with neighbouring chains. Shorter _Diatoma_ cell chains were persistently

entangled even when bent over by water flow. Model simulations suggest that long chains with a potentially larger interaction radius had higher chance to become entangled and to form

prominent arch-like structures as those observed in natural biofilms. After this threshold, arch-formation became more frequent eventually resulting in complex dome-shaped configurations.

Some of the arches pressed down to the substratum by other extending filaments were no longer able to move in the flow and a rather static structure emerged that reasonably well mimicked

observed patterns (Figure 2A). At the crest, simulations were run using the same input parameters (_Supplementary Methods_ and _Supplementary Table S1_) as in the trough scenario, except the

water flow velocity and its spatial distribution were altered. Simulations reflected natural conditions where unidirectional and faster flow bent growing _Diatoma_ chains over and pulled

them in one preferential direction. The flow environment prevented the development of an arch formation from the entanglement of neighbouring chains and ultimately the dome-like structures.

Rather, the long filamentous chains aligned themselves with the flow. Generally, interactions between chains were reduced compared to the trough, though inevitably, as chains grew longer,

entanglement and sticking did occur to form elongated dreadlock-like streamers that oscillated in the flow. DISCUSSION Diatoms greatly contribute to the biomass and structure of phototrophic

biofilms in streams and rivers where they are involved in key processes including whole-ecosystem primary production, autotrophic respiration and organic matter degradation22,23. Depending

on the local flow environment, these benthic biofilms develop complex architectures, which in turn can affect local hydrodynamics but also carbon and nutrient dynamics8,11,24. Understanding

mesoscale (millimetre range) biofilm morphogenesis and resulting architectures is necessary to better evaluate mass transfer and related phenomena in biofilms25,26. The model constructed in

this study, in conjunction with empirical observations, unravels the morphogenesis of phototrophic biofilms dominated by colony-forming diatoms as often encountered in pristine streams.

Embedded in the local hydrodynamics, a simple mechanical mass-spring model qualitatively simulated the growth and behaviour of _Diatoma_ chains and their assembly to higher complexity

structures. Numerical simulations illustrated how colonies and chains develop from single _Diatoma_ cells that randomly adhere to the substratum. Short evolving chains were characterized by

a relatively high flexural stiffness and were only marginally affected by hydrodynamics. However, as _Diatoma_ chains elongated, their flexibility and the flow momentum allowed their tips to

explore a larger territory, thereby increasing the probability to encounter and to stick to adjacent chains. It seems that this phase is a tipping point in the morphogenesis as, dependent

on the hydrodynamic environment, _Diatoma_ chains can now assemble into different morphologies depending on the hydrodynamic microenvironments at the crest and in the trough. At the crest,

adjacent _Diatoma_ chains entangle and, guided by predominantly unidirectional flow, form dreadlock-like streamers. Streamers are universal biofilm architectures that have been reported from

monospecies bacterial biofilm growing under turbulent27 and laminar10,28 flow, but also from complex stream biofilms containing bacteria, diatoms and EPS29. Elevated flow velocities at the

crest may improve nutrient flux and mass transfer, besides the mass transfer enhancement due to the oscillatory movement of streamers30,31. Only those filaments that are securely anchored

can flourish in this environment. However, this elevated shear stress is also likely to damage weaker streamers and may impede the attachment of unicellular organisms. In the trough,

morphogenesis results in networks with Y-shaped structures as basic elements. Similar structures were reported from cyanobacterial mats where motile _Pseudoanabena_ cells collide, align and

clump to produce intersecting ridges that surround areas with low cell density32. Furthermore, using a size-structured population model, Hödl et al. (2013) showed how short-range dispersal

of cells and coalescence of adjacent microbial clusters may result, depending on the prevailing direction of water flow, in networked or longitudinal structures in complex stream biofilms.

Our results now identify diatoms as key architects of these apparently universal biofilm structures. Their important role in guiding physical structure may explain in part why diatoms have

been so successful in colonizing most aquatic ecosystems1,33. Of major evolutionary advantage to _Diatoma_ is their encasement in a silica shell and capability to form chains, which may

improve nutrient supply, protect from grazing and counteract sinking in pelagic ecosystems2,4,33. Numerical simulations suggest that stiff diatom chains experience higher nutrient flux in a

turbulent flow environment than flexible chains2. Our simulations show that nascent and short _Diatoma_ chains bend less compared to longer chains. This would thus provide an advantageous

nutrient environment to these cells during initial and fast growth, as stiff chains may maintain a larger effective size, cover a larger territory and are likely to encounter more random

nutrient sources2. As further revealed by the simulations, elongated chains exhibit less resistance to the flow and bend more, which, according to Musielak _et al_.2, would induce a less

favourable nutrient environment. The entanglement into arch-like and Y-like structures counteracts the high flexibility of individual chains thereby potentially enhancing mass flux per cell

at this later stage of biofilm growth. Furthermore, our simulations suggest that _Diatoma_ structures, whether entangled in filamentous streamers or forming the more complex

three-dimensional scaffolds, reversibly respond to the hydrodynamic forces and thus exhibit rheological properties similar to the viscoelasticity of biofilm EPS34. This would be a further

advantage to the _Diatoma_ chains now forming the scaffold for further biofilm growth. Modelling revealed the importance of growth rate and sticking frequency on _Diatoma_ chain formation.

Spatial proximity of chains to one another at initialization affected whether and when the flow would bring these filaments together; careful adjustment of sticking probability was necessary

to ensure that chains did not become over-tangled. In fact, in nature, flow is likely to sometimes detach chains that are attached to one another or the substratum. In numerical models,

biofilm development is usually described by a balance between microbial growth and attachment (contributing to increase in biofilm volume) and biofilm detachment (leading to biomass loss)35.

This balance is essential when explaining the formation of different biofilm morphologies36. Implementation of detachment in future models should provide further insight into the effect of

physical dispersal on chain formation. Additional detail (e.g., processes such as sticking, microbial motility, selective attachment places, or specific cell-cell interactions) can also be

added using an individual-based modelling approach as the one developed in this study. Flow shapes not only architecture but also community composition37,38. It would thus also be of great

value to extend the model to include the effect of biodiversity on streamer and colony formation. Though diatom chains seem to form the structural scaffolding of phototrophic biofilms,

additional microorganisms have a large impact on growth and extension of colonies. At low flow, there may be higher diversity due to increased aggregation, with structure oscillations

increasing particle trapping39. At high flow, however, an increased flux of microorganisms may occur from the bulk liquid to the biofilm29. Extending the mass-spring framework to account for

differential attachment of microbes at the crest and trough should provide additional insight into community succession at different hydrodynamic conditions. METHODS HYDRODYNAMIC

ENVIRONMENT A streamside flume (length: 40 m) was constructed to capture the diversity of biofilms from various hydrodynamic environments and growth stages, mimicking a flow landscape

typical for streams with low submergence21. The experimental set-up has been described in detail previously11. Briefly, graded and periodically installed bedforms (bottom length: 1 m; width:

0.40 m; ascending slope: 0.75 m; descending slope: 0.25 m; maximum elevation: 0.08 m) were installed in the flume, which was continuously fed in a once-through mode with stream water

(Oberer Seebach); flow rate was adjusted to 2.25 L s−1 with an average flume-scale flow velocity of 0.08 m s−1; average residence time of water was 8 minutes in the flume. The crest and the

trough were selected as hydrodynamic extremes. High-resolution Acoustic Doppler Velocimetry (ADV, Nortek Vectrino, side-looking probe) was used to capture the three-dimensional flow velocity

over the bedforms. BIOFILM GROWTH Biofilms were grown on initially sterile glass slides that were ignited (450°C, 4 h) to remove organics40. Replicate glass slides were exposed at the crest

and in the trough of repeated bedforms. Growth of complex biofilms including bacteria, algae and non-living particles typically occurred within two weeks and reflects the communities in the

streamwater24,37,40. MASS-SPRING COMPUTATIONAL MODEL _Diatoma_ chains were modelled as a collection of masses connected by springs and exposed to model processes of: (1) movement and

deformation within the flow; (2) growth by division of _Diatoma_ cells; (3) collision between different chains; (4) attachment of new cells to the support material; and (5) sticking of the

chains to one another. General details are provided below; for more information, the reader is referred to the _Supplementary Methods_ . * 1 _Diatoma_ chain movement A _Diatoma_ chain is

modelled as an array of particles with mass, connected by three spring types, which build rigid cells, keep angles between cells and ensure the zigzag conformation of the chain (Figure 2b).

Chain movement is determined by the motion of each individual particle of that particular chain. The particle movement is governed by the resultant of all the forces acting on it, according

to Newton's laws. Each particle _i_ is characterised by position X_i_ = [_x__i_,_y__i_,_z__i_] and velocity V_i_ = [_v__xi_,_v__yi_,_v__zi_] vectors, which concatenated form a phase

space and the mass _m__i_. The first particle of a _Diatoma_ chain is irreversibly attached to the substratum and the other _n_ particles can move. For the whole set of _f_ chains, equations

of motion can be written in a compact vector form as the system of 6×_n_×_f_ ordinary differential equations (ODE). To construct the phase space, the forces acting on each particle were

computed and resolved into components in the three directions. Each particle has several different forces acting on it: drag F_D,i_ and lift F_L,i_ resulting from the applied force of

flowing water, gravity and buoyancy combined in F_G,i_, as well as elastic forces from the first- (F_E1,i_), second- (F_E2,i_) and third-order (F_E3,i_) springs, from collisions (F_C,i_) and

from sticking-springs (F_S,i_). The contributions of each of these forces were added to form the combined force F_i_ used in the equation of motion (1). A representation of the forces

acting on a particle is given in Figure 3B and more information on force computations is in the _Supplementary Methods_ . * 2 Chain growth Chain growth in length is due to reproduction of

_Diatoma_ cells. These cells are inserted in the _Diatoma_ zigzag chain over time. This process was split into two phases in the model: cell ageing and cell division. No cell growth

limitation by light or nutrients was taken into account. Therefore, the filament chain growth follows an exponential relationship. * 3 Chain collision Movement of the chain necessitated

implementation of a collision detection and response algorithm to ensure that, during movement, each chain does not pass through itself, the other chains, or the substratum. A collision

response is triggered when the shortest distance between each two segments lies below a threshold value (i.e., twice the _Diatoma_ cell radius). The response takes the form of a repulsive

force F_C_ applied weighted to the two ends of each colliding cell. * 4 Chain attachment Attachment is the process in which cells stick to the substratum. Attachment involved adding a new

chain consisting of three particles (i.e., two _Diatoma_ cells connected under the characteristic angle) to a randomly chosen position (standard uniform distribution in both _L__x_ and

_L__y_) on the substratum base. Such an event was implemented to occur at the end of each growth step, provided that the maximum number of daily attachments was not exceeded (one at the

ridge, two in the valley). These values were based on measured attachment frequencies at the crest and in the trough (see model parameters in the _Supplementary Methods_ ). * 5 Chain

sticking To realistically simulate sticking between chains, the assumption was made that within a given growth time step, sticking occurs in 1 in 10,000 collisions (standard uniform

distribution), with a maximum number of three sticking events per movement time step. These values result in visually realistic colony architecture formation for the given system size and

flow parameters. Sticking results in the creation of an elastic spring (FS) between the two particles that have collided, which keeps the two sticking chains together. MODEL SOLUTION

Modelling movement involved the solution of the ordinary differential equations for each particle in the system by an explicit eighth-order Dormand-Prince routine41. Integration results were

saved at intervals _Δt__m,s_ within the movement time step _Δt__m_. In order to visualize the results, the freely available ray-tracing software Persistance of Vision (POV-Ray,

www.povray.org) was used. REFERENCES * Bowler, C., Vardi, A. & Allen, A. E. Oceanographic and biogeochemical insights from diatom genomes. Ann. Rev. Mar. Sci. 2, 333–365 (2010). Article

  Google Scholar  * Musielak, M. M., Karp-Boss, L., Jumars, P. A. & Fauci, L. J. Nutrient transport and acquisition by diatom chains in a moving fluid. J. Fluid. Mech. 638, 401–421

(2009). Article  CAS  ADS  MathSciNet  Google Scholar  * Jumars, P. A., Trowbridge, J. H., Boss, E. & Karp-Boss, L. Turbulence-plankton interactions: a new cartoon. Mar. Ecol-Evol.

Persp. 30, 133–150 (2009). Article  ADS  Google Scholar  * Young, A. M., Karp-Boss, L., Jumars, P. A. & Landis, E. N. Quantifying diatom aspirations: mechanical properties of

chain-forming species. Limnol. Oceanogr. 57, 1789–1801 (2012). Article  ADS  Google Scholar  * Gerbersdorf, S. U., Jancke, T., Westrich, B. & Paterson, D. M. Microbial stabilization of

riverine sediments by extracellular polymeric substances. Geobiology 6, 57–69 (2008). CAS  PubMed  Google Scholar  * Decho, A. W. Microbial biofilms in intertidal systems: an overview. Cont.

Shelf Res. 20, 1257–1273 (2000). Article  ADS  Google Scholar  * Battin, T. J. et al. Biophysical controls on organic carbon fluxes in fluvial networks. Nat. Geosci. 1, 95–100 (2008).

Article  CAS  ADS  Google Scholar  * Battin, T. J., Kaplan, L. A., Denis Newbold, J. & Hansen, C. M. Contributions of microbial biofilms to ecosystem processes in stream mesocosms.

Nature 426, 439–442 (2003). Article  CAS  ADS  Google Scholar  * Stoodley, P., Lewandowski, Z., Boyle, J. D. & Lappin-Scott, H. M. Oscillation characteristics of biofilm streamers in

turbulent flowing water as related to drag and pressure drop. Biotechnol. Bioeng. 57, 536–544 (1998). Article  CAS  Google Scholar  * Rusconi, R., Lecuyer, S., Autrusson, N., Guglielmini, L.

& Stone, H. A. Secondary flow as a mechanism for the formation of biofilm streamers. Biophys. J. 100, 1392–1399 (2011). Article  CAS  ADS  Google Scholar  * Hödl, I. et al. Biophysical

controls on cluster dynamics and architectural differentiation of microbial biofilms in contrasting flow environments. Environ. Microbiol. (2013). 10.1111/1462-2920.12205. * Parsek, M. R.

& Tolker-Nielsen, T. Pattern formation in _Pseudomonas aeruginosa_ biofilms. Curr. Opin. Microbiol. 11, 560–566 (2008). Article  CAS  Google Scholar  * Taşkiran, H. D. & Gūdūkbay, U.

Physically based simulation of hair strips in real time. Proc. 13th Central Europe Conf. Computer Graphics, Visualization and Computer Vision, 153–156 (2005). * Windisch, B., Bray, D. &

Duke, T. Balls and chains - a mesoscopic approach to tethered protein domains. Biophys. J. 91 (2006). * Provot, X. Deformation constraints in a mass-spring model to describe rigid cloth

behavior. Graphics Interface, 147–155 (1995). * Janulevicius, A., van Loosdrecht, M. C. M., Simone, A. & Picioreanu, C. Cell Flexibility Affects the Alignment of Model Myxobacteria.

Biophys. J. 99, 3129–3138 (2010). Article  CAS  ADS  Google Scholar  * Harvey, C. W. et al. Study of elastic collisions of _Myxococcus__xanthus_ in swarms. Phys. Biol. 8 (2011). Article  ADS

  Google Scholar  * Tamulonis, C., Postma, M. & Kaandorp, J. Modeling filamentous cyanobacteria reveals the advantages of long and fast trichomes for optimizing light exposure. PLoS One

6 (2011). * Dillon, R., Fauci, L., Fogelson, A. & Gaver, D. Modeling biofilm processes using the immersed boundary method. J. Comput. Phys. 129, 57–73 (1996). Article  CAS  ADS  Google

Scholar  * Alpkvist, E. & Klapper, I. Description of mechanical response including detachment using a novel particle model of biofilm/flow interaction. Water Sci. Technol. 55, 265–273

(2007). Article  CAS  Google Scholar  * Singer, G. et al. Microcosm design and evaluation to study microbial biofilms. Limnol. Oceanogr. Meth. 4, 436–447 (2006). Article  Google Scholar  *

Cummins, K. W. Structure and function of stream ecosystems. BioScience 24, 632–641 (1974). Article  Google Scholar  * Danger, M. et al. Benthic algae stimulate leaf litter decomposition in

detritus-based headwater streams: a case of aquatic priming effect? Ecology 94, 1604–1613 (2013). Article  Google Scholar  * Singer, G., Besemer, K., Schmitt-Kopplin, P., Hödl, I. &

Battin, T. J. Physical heterogeneity increases biofilm resource use and its molecular diversity in stream mesocosms. PLoS One 5 (2010). * Milferstedt, K., Pons, M. N. & Morgenroth, E.

Analyzing characteristic length scales in biofilm structures. Biotechnol. Bioeng. 102, 368–379 (2009). Article  CAS  Google Scholar  * Wagner, M., Taherzadeh, D., Haisch, C. & Horn, H.

Investigation of the mesoscale structure and volumetric features of biofilms using optical coherence tomography. Biotechnol. Bioeng. 107, 844–853 (2010). Article  CAS  Google Scholar  *

Stoodley, P., Debeer, D. & Lewandowski, Z. Liquid flow in biofilm systems. Appl. Environ. Microbiol. 60, 2711–2716 (1994). CAS  PubMed  PubMed Central  Google Scholar  * Drescher, K.,

Shen, Y., Bassler, B. L. & Stone, H. A. Biofilm streamers cause catastrophic disruption of flow with consequences for environmental and medical systems. Proc. Natl. Acad. Sci. USA. 110,

4345–4350 (2013). Article  CAS  ADS  Google Scholar  * Besemer, K. et al. Biophysical controls on community succession in stream biofilms. Appl. Environ. Microbiol. 73, 4966–4974 (2007).

Article  CAS  Google Scholar  * Taherzadeh, D., Picioreanu, C. & Horn, H. Mass transfer enhancement in moving biofilm structures. Biophys. J. 102, 1483–1492 (2012). Article  CAS  ADS 

Google Scholar  * Taherzadeh, D. et al. Computational study of the drag and oscillatory movement of biofilm streamers in fast flows. Biotechnol. Bioeng. 105, 600–610 (2010). Article  CAS 

Google Scholar  * Shepard, R. N. & Sumner, D. Y. Undirected motility of filamentous cyanobacteria produces reticulate mats. Geobiology 8, 179–190 (2010). Article  CAS  Google Scholar  *

Kooistra, W. H. et al. Global diversity and biogeography of Skeletonema species (bacillariophyta). Protist 159, 177–193 (2008). Article  CAS  Google Scholar  * Flemming, H. C. &

Wingender, J. The biofilm matrix. Nat. Rev. Microbiol. 8, 623–633 (2010). Article  CAS  Google Scholar  * Wanner, O. & Reichert, P. Mathematical modeling of mixed-culture biofilms.

Biotechnol. Bioeng. 49, 172–184 (1996). Article  CAS  Google Scholar  * Van Loosdrecht, M. C. M., Picioreanu, C. & Heijnen, J. J. A more unifying hypothesis for biofilm structures. FEMS

Microbiol. Ecol. 24, 181–183 (1997). Article  CAS  Google Scholar  * Besemer, K., Singer, G., Hödl, I. & Battin, T. J. Bacterial community composition of stream biofilms in spatially

variable-flow environments. Appl. Environ. Microbiol. 75, 7189–7195 (2009). Article  CAS  Google Scholar  * Stoodley, P., Sauer, K., Davies, D. G. & Costerton, J. W. Biofilms as complex

differentiated communities. Annu. Rev. Microbiol. 56, 187–209 (2002). Article  CAS  Google Scholar  * Rickard, A. H., McBain, A. J., Stead, A. T. & Gilbert, P. Shear rate moderates

community diversity in freshwater biofilms. Appl. Environ. Microbiol. 70, 7426–7435 (2004). Article  CAS  Google Scholar  * Hödl, I. et al. Voronoi tessellation captures very early

clustering of single primary cells as induced by interactions in nascent biofilms. PLoS One 6 (2011). Article  ADS  Google Scholar  * Press, W. H., Teukolsky, S. A., Vetterling, W. T. &

Flannery, B. P. Numerical Recipes: the Art of Scientific Computing. 3rd edn, (Cambridge University Press, 2007). Download references ACKNOWLEDGEMENTS CP acknowledges the financial support by

the Netherlands Organization for Scientific Research (NWO, VIDI grant 864.06.003). TJB was supported by the Austrian Science Fund (START Y420-B17) and IH by the PhD School “Symbiosis and

Biotic Interactions”, University of Vienna. AUTHOR INFORMATION AUTHORS AND AFFILIATIONS * Department of Biotechnology, Delft University of Technology, Julianalaan 67, Delft, 2828, BC, The

Netherlands K. Celler & C. Picioreanu * Molecular Biotechnology, Institute of Biology Leiden, Sylvius Laboratories, Leiden University, Sylviusweg 72, Leiden, 2333, BE, The Netherlands K.

Celler * Department of Limnology and Oceanography, University of Vienna, A-1090, Vienna, Austria I. Hödl & T. J. Battin * Delft University of Technology, Faculty of Civil Engineering

and Geosciences, Delft University of Technology, P.O. Box 5048, 2600, GA, Delft, The Netherlands A. Simone Authors * K. Celler View author publications You can also search for this author

inPubMed Google Scholar * I. Hödl View author publications You can also search for this author inPubMed Google Scholar * A. Simone View author publications You can also search for this

author inPubMed Google Scholar * T. J. Battin View author publications You can also search for this author inPubMed Google Scholar * C. Picioreanu View author publications You can also

search for this author inPubMed Google Scholar CONTRIBUTIONS K.C., A.S. and C.P. developed the mathematical model. I.H. designed and performed experiments and the conceptual model. T.B. and

C.P. arranged funding, designed experiments and supervised all work. K.C., T.B. and C.P. wrote the manuscript. ETHICS DECLARATIONS COMPETING INTERESTS The authors declare no competing

financial interests. ELECTRONIC SUPPLEMENTARY MATERIAL SUPPLEMENTARY INFORMATION Supplementary Methods, Tables and Figures SUPPLEMENTARY INFORMATION Supplementary Material Video SV1

SUPPLEMENTARY INFORMATION Supplementary Material Video SV2 RIGHTS AND PERMISSIONS This work is licensed under a Creative Commons Attribution-NonCommercial-NoDerivs 3.0 Unported License. To

view a copy of this license, visit http://creativecommons.org/licenses/by-nc-nd/3.0/ Reprints and permissions ABOUT THIS ARTICLE CITE THIS ARTICLE Celler, K., Hödl, I., Simone, A. _et al._ A

mass-spring model unveils the morphogenesis of phototrophic _Diatoma_ biofilms. _Sci Rep_ 4, 3649 (2014). https://doi.org/10.1038/srep03649 Download citation * Received: 06 September 2013 *

Accepted: 11 December 2013 * Published: 13 January 2014 * DOI: https://doi.org/10.1038/srep03649 SHARE THIS ARTICLE Anyone you share the following link with will be able to read this

content: Get shareable link Sorry, a shareable link is not currently available for this article. Copy to clipboard Provided by the Springer Nature SharedIt content-sharing initiative