Soliton bursts and deterministic dissipative kerr soliton generation in auxiliary-assisted microcavities

ABSTRACT Dissipative Kerr solitons in resonant frequency combs offer a promising route for ultrafast mode-locking, precision spectroscopy and time-frequency standards. The dynamics for the

dissipative soliton generation, however, are intrinsically intertwined with thermal nonlinearities, limiting the soliton generation parameter map and statistical success probabilities of the

solitary state. Here, via use of an auxiliary laser heating approach to suppress thermal dragging dynamics in dissipative soliton comb formation, we demonstrate stable Kerr soliton singlet

formation and soliton bursts. First, we access a new soliton existence range with an inverse-sloped Kerr soliton evolution—diminishing soliton energy with increasing pump detuning. Second,

we achieve deterministic transitions from Turing-like comb patterns directly into the dissipative Kerr soliton singlet pulse bypassing the chaotic states. This is achieved by avoiding

subcomb overlaps at lower pump power, with near-identical singlet soliton comb generation over twenty instances. Third, with the red-detuned pump entrance route enabled, we uncover unique

spontaneous soliton bursts in the direct formation of low-noise optical frequency combs from continuum background noise. The burst dynamics are due to the rapid entry and mutual attraction

of the pump laser into the cavity mode, aided by the auxiliary laser and matching well with our numerical simulations. Enabled by the auxiliary-assisted frequency comb dynamics, we

demonstrate an application of automatic soliton comb recovery and long-term stabilization against strong external perturbations. Our findings hold potential to expand the parameter space for

ultrafast nonlinear dynamics and precision optical frequency comb stabilization. SIMILAR CONTENT BEING VIEWED BY OTHERS DISSIPATIVE SOLITON GENERATION AND REAL-TIME DYNAMICS IN

MICRORESONATOR-FILTERED FIBER LASERS Article Open access 12 October 2022 ZERO DISPERSION KERR SOLITONS IN OPTICAL MICRORESONATORS Article Open access 13 August 2022 RETIMING DYNAMICS OF

HARMONICALLY MODE-LOCKED LASER SOLITONS IN A SELF-DRIVEN OPTOMECHANICAL LATTICE Article Open access 02 February 2025 INTRODUCTION Dissipative Kerr solitons (DKS) can be obtained in optical

cavities through the double balancing of cavity loss with third-order nonlinear parametric amplification and cavity dispersion with self-phase modulation (SPM)1,2,3. In particular, DKS

generated in high finesse microresonators associated with a Kerr frequency comb has attracted considerable interest, as it simultaneously gives rise to ultrashort mode-locked pulses4,5,6 and

broadband comb spectra7,8,9,10 with good intrinsic stabilization potential11 and a smooth envelope12. Moreover, DKS microcombs not only exhibit abundant physical

dynamics13,14,15,16,17,18,19,20,21,22,23,24,25,26 but also demonstrate immense practical prospects ranging from high capacity fiber transmission27,28, an ultra-stable microwave source29, and

a photonic frequency synthesizer30, to precision laser metrology and spectroscopy31,32,33,34,35. The robust generation of DKS, especially the single soliton state, however, remains

difficult due to the sizable cavity thermal nonlinearity1,36, which prevents the pump laser from entering the parameter space where the dual-balanced solitary waveforms exist. The current

strategy used for DKS formation is based on the fast scanning of pump laser frequency to overtake the slower dragged thermal response in a rapidly changing intracavity waveform and stopping

the pump as close to the optimal condition as possible6,15,37,38,39,40,41. This scheme sets the microresonator to a delicate thermal equilibrium with complex detuning conditions of the

intracavity light fields;1,15 consequently, one requires rigidly conducted pump frequency-sweeping39,40,41 and “power kicking”5,38, during which precision control of the soliton state is

cumbersome38,40. Moreover, such a fast pump scanning approach contains an intrinsic prerequisite that, to keep the cavity in thermal equilibrium, the intracavity light fields must sustain

effective blue-detuning even after the pump laser enters the red-detuning resonance region1,40. This prerequisite results in high pump power operation and, thereby, a chaotic stage prior to

accessing the low-noise soliton states42,43,44. The resulting DKS pulse number and its comb envelope thus vary from measurement to measurement. Consequently, thermal compensation approaches

have been investigated, such as cavity optomechanics with dual lasers45 and stabilization of the cavity mode spacing46. Here, we demonstrate, via an auxiliary laser heating approach, an

expanded operating phase space for the nonlinear transitions of the Kerr frequency comb along with unique dynamics. Through separation of the cavity thermal nonlinearities from the Kerr

dynamics, we first uncover an existence range in the inverse-sloped Kerr soliton evolution featuring a diminishing soliton energy with increasing pump detuning, arising from a unique balance

between soliton peak power and pulse width. Second, we report deterministic transitions from Turing-like comb patterns directly into a dissipative Kerr soliton singlet pulse, avoiding the

subcomb overlaps and thereby bypassing the chaotic states in the formation dynamics. Third, we illustrate dissipative Kerr soliton bursts arising from background noise via the red-detuning

pump entrance. Aided by these new findings, we implement an automatic soliton recovery algorithm against strong external perturbations. RESULTS STABLE DKS GENERATION VIA AUXILIARY LASER

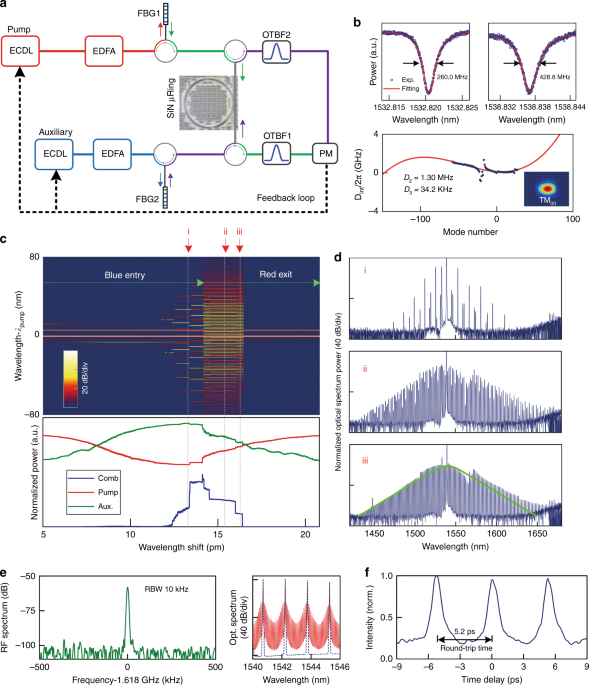
HEATING Figure 1a illustrates our experimental setup. A Si3N4 microring cavity with a width-height cross-section of 2000 × 800 nm2 and a loaded _Q_-factor of ≈500,000 is utilized for

frequency comb generation. More than ninety resonant frequencies of the TM00 mode are recorded via a Mach–Zehnder interferometer (MZI)-based optical sampling technique47 from which the

cavity group velocity dispersion (GVD) is retrieved. The measured dispersion matches well with finite-difference time-domain (FDTD) numerical simulations, as shown in Fig. 1b, with a

second-order GVD (_D__2_) of 1.30 MHz and third-order dispersion (_D__3_) of 34.2 kHz obtained. GVD discontinuity of the TM00 mode caused by mode-crossing with another spatial mode family is

observed4,12, the influence of which will be discussed in the below sections. Our approach uses two external cavity diode lasers (ECDL), which are amplified and launched into the

microcavity from opposite directions (Fig. 1a). First, an auxiliary laser (_E_aux) is frequency tuned into a resonance (≈1532.8 nm in our experiment) following the traditional self-thermal

locking trajectory and is stopped near the resonance peak but still kept blue-detuned. Subsequently, a pump laser (_E_pump) is tuned into another resonance (≈1538.8 nm) in the

counter-propagating direction. Due to the effect of _E_aux, the evolution of _E_pump is significantly modified from the conventional pathway. In particular, as _E_pump is tuned into

resonance from the blue side, all the resonances are heated and thermally pushed to longer wavelengths, which displaces _E_aux from its resident resonance and cools the microcavity in

counter-balance. Likewise, when _E_pump leaves the cavity resonance from the red side and cools the cavity, _E_aux will re-enter its resident resonance and in turn heat the cavity (see

Supplementary Information Section I). By aptly configuring the power and frequency for _E_aux, the heat flow caused by _E_pump can be largely balanced out, keeping the cavity temperature and

all cavity resonances approximately unchanged. This allows the pump laser to be stably tuned across the entire resonance with minimized thermal behavior. Furthermore, since _E_aux and

_E_pump are counter-propagating light waves with different detunings, the Kerr-nonlinearity cross-phase modulation (XPM) between them induces a slowly varying nonlinear detuning offset48,

which retards the change in the effective pump detuning (similar to thermal counter-dragging) and in turn increases the detuning range wherein DKS can be accessed (see Supplementary

Information Section VIII). As shown in Fig. 1c, by implementing a dual-driven scheme, the experimentally measured power transmissions, _E_pump and _E_aux, clearly illustrate their

counter-balanced contributions to the cavity thermal behavior. We observe that when the pump traverses from the blue-detuning (_λ_i) to the red-detuning (_λ_iii) regime, stable comb spectra

can be formed, as shown in Fig. 1d-(i)–d-(iii). Specifically, when the _E_pump is blue-detuned (_λ_i), subcombs with well separated line doublets (i.e., spacing multiple FSR) are

generated42, as shown in Fig. 1d. When the pump enters the red-detuned regime (_λ_ii), the oscillator achieves gap-free single FSR comb spectra resembling multiple DKS waveforms. Upon

further tuning the pump toward the red-sided regime, we observe step-like discrete soliton annihilations, and eventually, a single soliton microcomb with a _sech__2_ envelope is achieved

(_λ_iii). The RF spectra are consistently at the noise floor for all three comb states. Moreover, the beat note of the single DKS comb is measured via a cross-phase modulation method

(detailed in Supplementary Information Section II). As shown in Fig. 1e, the beat note is much narrower than the pump laser linewidth (≈500 kHz), confirming the good coherence of the DKS

microcomb. We also conducted second-harmonic generation (SHG)-based autocorrelation measurement of the single DKS comb. As displayed in Fig. 1f, clear pulses are obtained with a temporal

width of ≈650 fs and period of 5.2 ps, consistent with the cavity round-trip time and confirming singlet DKS operation (detailed in Supplementary Information Section IV). Our

auxiliary-driven approach relaxes the prior fast sweeping rate requirement for the pump laser for DKS formation, allowing ease in setting the various DKS states at different detunings for

dynamical exploration. EXISTENCE OF A DKS REGIME SUPPORTING DIMINISHING SOLITON PULSES A noteworthy feature of the auxiliary-assisted DKS evolution is observed in Fig. 1c. Specifically the

step-like comb power evolution (blue line) exhibits an inverse negative slope as a function of pump detuning. In other words, as the pump detuning increases in this experiment, the overall

DKS energy decreases, revealing a diminishing soliton regime. Such regimes were absent in prior DKS generation schemes realized via fast-pump-tuning, which always has an increasing

intracavity energy with larger pump detuning (i.e., positive-slope soliton stairs) to maintain soliton self-thermal-locking1,12,15,38,39. Along with the diminishing soliton energy with pump

detuning, in our studies, we also observed appreciable relaxation of the pump power requirement for DKS formation. As shown in Fig. 3a, a single DKS comb state was generated with 0.15 W of

on-chip pump power, which is much smaller than prior DKS generation with a similar quality factor6,24. To discern these new phenomena, we conduct a Lugiato-Lefever equation (LLE) simulation

using our experimental parameters (detailed in the Materials and Methods). Figure 2a compares the experimental and theoretical pump and comb power evolutions, which agree well with each

other; both exhibit inverse-slope comb power steps. To verify DKS existence in this new range, in Fig. 2b, we illustrate the calculated comb power versus pump detuning (Δ_P_comb/Δ_δ_) in a

wide parameter space using the pulse seeding method14,26. At a relatively high pump power, DKS evolution has a typical positive slope as shown in the yellow to orange region. In contrast, at

lower pump powers, a new diminishing soliton regime with an inverse-slope is clearly identified by the gray to black regions in this map. The underlying physical mechanism for different DKS

regimes lies in the subtle variation of the DKS pulse evolutions. As shown in Fig. 2c, with the conventional higher pump power, the increase in soliton peak power is more prominent than the

decrease in soliton pulse width, so that the overall pulse energy increases with pump detuning. In contrast, when the pump power is lowered with our auxiliary laser heating approach, the

order of importance between the soliton peak power and pulse width is reversed, and the overall soliton energy decreases with increasing detuning (complementary measurements and analytical

formula are detailed in Supplementary Information Sections V and VI). It is notable that this new diminishing soliton regime with a relaxed extrinsic pump power requirement can help to

expand the DKS existence range in relatively low-finesse microcavities7, supporting octave-spanning Kerr combs with ultrashort DKS pulse widths and low energies40, enabling low repetition

rate DKS in long microcavities49, and allowing deterministic DKS formation, as presented below. DETERMINISTIC DKS FORMATION WITHOUT CHAOTIC STAGES In addition to an expanded existence

regime, the auxiliary-assisted microcavity allows the robust evolution of the frequency comb into the singlet DKS state. Figure 3a shows twenty example repeated measurements of the

time-trace for the comb power evolution, each of which evolves to a singlet DKS. This phenomenon is distinct from prior demonstrations using either fast-swept pump or power-kicking

schemes5,15,38 in which the DKS state has a statistical success probability, and the eventual DKS pulse numbers are not deterministically controlled. As reported in recent studies50,51, this

phenomenon relies on the mechanism that the mode interaction-induced GVD discontinuity can suppress or enhance individual comb lines18,50, giving rise to an oscillatory background attached

to each DKS, akin to the effect of Kelly sidebands in mode-locked fiber lasers52. Such oscillating pump backgrounds facilitate mutual soliton interferences14, and finally only one last DKS

survives as the pump detuning approaches the maximum value (i.e., the pump power approaches a minimum value) before the comb collapses50. To verify this phenomenon, we conduct LLE modeling

using the measured GVD data that include discontinuities from local mode interactions (blue dots in Fig. 1b)53. As shown in Fig. 3a (bottom left panel), twenty independent LLE simulations

initiated by random noise seeds all result in a singlet DKS state, agreeing well with our measurements. Meanwhile, in Fig. 3a, we observed that for each measurement, the comb power evolution

paths to the singlet DKS state still undergo random changes. This randomness arises from the overlaps of the subcomb family during Kerr comb generation, which includes a chaotic stage in

the intracavity waveform formation and sets a statistically random initial condition for the subsequent DKS convergence1, as illustrated in Fig. 3b. Usually in the fast-pump-tuning scheme,

strong pump intensity is required to maintain self-thermal locking of the DKS state, and the dynamical route has to go through this chaotic regime44,54. Our auxiliary laser heating approach

reduces the requirement on the pump intensity for DKS formation, as shown in Fig. 2b. This allows us to further reduce the pump intensity and explore the direct transition from a stable

Turing pattern (i.e., primary comb lines) to the low-noise DKS state, avoiding the chaotic stage. Figure 3a (top right panel) shows the measured comb power evolution to the single DKS state

at 0.8 W of launched pump power (0.15 W of on-chip power, considering 7.0 dB attenuation during pump delivery). Strikingly, almost identical comb power trances are experimentally recorded in

twenty independent DKS transition measurements (minor discrepancies are due to laser wavelength sweep repeatability fluctuations), all of which result in a singlet DKS without exception.

Such dynamics are also captured in the LLE modeling, as shown in the lower right panel of Fig. 3a, confirming the deterministic formation of the single DKS comb without intermediate chaos.

Figure 3b shows the numerically resolved spectral and temporal evolution of the DKS comb with a pump power of 0.15 W. We observe that, due to the relatively low pump power, the Kerr comb

spectrum exhibits no subcomb overlap when the pump is blue-detuned. Subsequently the pump directly enters the red-detuning side without triggering subcomb spectral overlap. Thus, this

intrinsically allows the temporal Turning pattern to directly and deterministically transit into the DKS state without a chaotic intermediate stage (the latter is typically formed from the

subcomb dynamics). As the pump detuning further increases, multiple solitons step-wise annihilate and the singlet DKS state is reached aided by the GVD discontinuity50,51. OBSERVATION OF DKS

BURSTS WITH RED-DETUNED PUMP ENTRANCE For conventional Kerr comb generation, the pump laser always enters cavity resonance from the blue-detuning regime so as to acquire self-thermal

locking36. As discussed above, the auxiliary laser heating scheme dispenses with canonical self-thermal locking and allows red-side pump entrance. This is demonstrated in Fig. 4a; we first

configure a blue-detuned auxiliary laser and then let the pump laser enter the cavity mode from the red-detuning regime. Intriguingly, we observe sharp _DKS burst_ dynamics directly from the

continuum background noise (_λ_i) in which a multiple soliton comb state with low RF noise is generated (Fig. 4b). To validate the soliton nature of the burst intracavity waveform, we then

tune the pump frequency out of resonance from the red side and, as expected, typical step-like soliton annihilations are detected (_λ_ii and _λ_iii). Figure 4c displays the fast DKS burst

dynamics measured with a real-time oscilloscope; it is seen that the burst process is completed in approximately 50 ns, within which the cavity thermal response and pump frequency tuning are

negligible, indicating the spontaneous nature of the DKS burst. LLE modeled burst dynamics illustrate remarkable agreement with experimental traces (Fig. 4c), allowing us to understand the

detailed kinetics. In particular, when the pump laser enters the cavity from the red-detuning regime, SPM incurred by the increasing intracavity pump power redshifts all the cavity

resonance, letting the cavity resonance and pump laser move towards each other. Resultantly, the pump laser builds up quickly and triggers Kerr comb generation after reaching the parametric

oscillation threshold. Meanwhile, the formation of Kerr comb lines causes a decrease in the intracavity pump power and a concomitant SPM resonance redshift; conversely, the pump laser leaves

the resonance from the red-detuning side, causing an iterative decrease in pump power. That is, the pump laser goes in and out of the cavity mode as a spontaneous chain reaction (since the

pump frequency is fixed), leaving only an excited Kerr comb waveform, which spontaneously develops into DKS pulses as the pump eventually stabilizes in the red-detuning regime. Moreover, LLE

modeled intracavity dynamics (Fig. 4d) give a clear visualization of the DKS burst mechanism: as the pump enters from the red-detuning side, even slight fluctuations within the pump

background (e.g., caused by GVD discontinuity) can ignite the chain reaction pump movement, causing sudden soliton appearance from the seemingly flat pump background. DISCUSSION DKS RECOVERY

AGAINST EXTERNAL PERTURBATIONS Enabled by a red-pump-entry spontaneous DKS burst and deterministic generation, Fig. 5 demonstrates the effective singlet DKS recovery against strong external

perturbations. As noted in prior studies, the DKS state can be vulnerable to pump laser instabilities including frequency noise, frequency drift and power fluctuations, requiring either

comb power or pump detuning feedback11,37,46,55. To enable long-term operation for the singlet DKS, we thus measure the total comb power as an error signal to control the auxiliary laser

wavelength for protected recovery of the DKS state against external variations. We implement a physical control algorithm as follows: when the comb power becomes smaller than a preset value

corresponding to the singlet DKS state, we assume that the target state is lost; then, the auxiliary laser is tuned closer to the resonance peak while thermally red-shifting all resonances,

and correspondingly, we let the pump re-enter from the red-detuned regime and trigger a DKS burst. Conversely, on the other side, when multiple solitons are regenerated with higher power

than that associated with the singlet DKS, we decrease the auxiliary laser wavelength to blue-shift the cavity resonance and, correspondingly, let the pump leave the resonance until the

singlet DKS comb power is restored. Figure 5 illustrates the experimentally measured singlet DKS recovery dynamics, which are triggered by two mechanical hard vibrations on the pump and

auxiliary lasers, respectively (labelled as events “1” and “2”), and two ambient acoustic disturbances (labelled as events “3” and “4”) – each of which are severe enough to drop the

operating DKS microcomb. To illustrate the singlet DKS recovery, the wavelength shift of the auxiliary laser is simultaneously read out, as shown as the black line in Fig. 5. The pump,

auxiliary and comb powers are shown as the red, green and blue lines, respectively. We observe that the singlet DKS comb state, characterized by the comb power, is successfully recovered

from each of the four externally enforced perturbation events, with the close-up slow-time dynamics of the fourth event shown in Fig. 5b. When a strong acoustic shock is applied to both the

pump and auxiliary lasers in event “4”, the comb power shows a fast decrease, indicating the collapse of all DKSs. At this stage, the cavity modes do not go back to their cold resonances due

to the presence of the auxiliary laser that resides on the blue-detuned regime; thus, the resonances remain approximately unmoved. Subsequently, based on the negative feedback recovery

algorithm, the decreased comb power leads to an increase in the wavelength of the auxiliary laser (black curve in Fig. 5), which thermally redshifts all cavity resonances and drives the pump

laser onto resonance again from the red-detuned side, thus causing the spontaneous DKS burst to recover the comb. The auxiliary laser wavelength is then automatically decreased again to

regain singlet DKS operation. We note that the auxiliary laser wavelength shift is all within 2 pm for successful DKS recovery, highlighting the efficacy of our recovery approach. We test

our spontaneous burst and deterministic DKS approach against different perturbation strengths, and in each instance, the singlet DKS comb is recovered as long as the auxiliary laser keeps

its thermal lock on the microcavity. In conclusion, assisted by an auxiliary-laser, we demonstrated for the first time the separation of thermal nonlinearity from Kerr nonlinearity in

microcavities. Counterbalancing the thermal nonlinearity, we uncover unique stable DKS formation routes in an expanded existence range featuring inverse-sloped soliton stairs. We also

observed a direct transition from Turing-like patterns into a DKS state avoiding chaotic stages, enabled by the use of unprecedentedly low (normalized) pump power. Furthermore, via a

red-detuned pump entrance, we demonstrated the existence of DKS bursts in which the soliton state is formed at the first stable instance directly through an instantaneous parametric

modulation instability state. With the expanded existence range and precise deterministic control of the DKS states, we illustrate the robustness of our singlet DKS state with the

application of dynamical feedback recovery against external perturbations in the frequency comb. This study enriches the physical understanding of dynamical Kerr solitons in dissipative

nonlinear cavities and provides a new architecture for mesoscopic mode-locked frequency combs. MATERIALS AND METHODS THEORETICAL MODEL FOR NUMERICAL SIMULATIONS The LLE model with a cavity

thermal effect and auxiliary laser is expressed as follows:14,56 $$\begin{array}{l}T_R\frac{{\partial E_{\mathrm{comb}}(t,\tau )}}{{\partial t}} = \left[ { - \alpha - i\left( {\delta

_{\mathrm{{pump}}} - \delta _{\mathrm{thermal}}} \right) + iL\mathop {\sum}\limits_{k \ge 2} \frac{{\beta _k}}{{k!}}\left( {i\frac{\partial }{{\partial \tau }}} \right)^k}\right.\\\left.+

i\gamma L\left( {\left| {E_{\mathrm{comb}}} \right|^2{\mathrm{ + 2}}P_{\mathrm{aux}}} \right) \right]E_{\mathrm{comb}}(t,\tau ) + \sqrt \theta E_{\mathrm{pump}}^{in}\end{array}$$ (1)

$$\begin{array}{l}T_R\frac{{\partial E_{\mathrm{aux}}(t,\tau )}}{{\partial t}} = \left[ { - \alpha - i\left( {\delta _{\mathrm{aux}} - \delta _{\mathrm{thermal}}} \right) + iL\mathop

{\sum}\limits_{k \ge 2} \frac{{\beta _k}}{{k!}}\left( {i\frac{\partial }{{\partial \tau }}} \right)^k}\right.\\\left.+ i\gamma L\left( {\left| {E_{\mathrm{aux}}} \right|^2 +

2P_{\mathrm{comb}}} \right) \right]E_{\mathrm{aux}}(t,\tau ) + \sqrt \theta E_{\mathrm{aux}}^{in}\end{array}$$ (2) $$\frac{{\partial \delta _{{\mathrm{thermal}}}}}{{\partial t}} =

\frac{{\mathrm{1}}}{{C_p}}\left[ {\alpha _T\frac{{\mathrm{Q}}}{{Q_{abs}}}\left( {P_{\mathrm{comb}} + P_{\mathrm{aux}}} \right) - K\delta _{{\mathrm{thermal}}}} \right]$$ (3) In Eq. (2), _T_R

is the round-trip time of the Si3N4 microcavity; _t_ is the slow time describing the evolution of the intracavity comb field _E_comb (_t_, _τ_) and auxiliary laser field _E_aux (_t_, _τ_)

at the scale of the cavity photon lifetime; _τ_ is the fast time defined in a reference frame moving at the light group velocity in the cavity; _α_ _=_ 0.009 is the cavity decay per

roundtrip; and _δ_pump and _δ_aux are the detunings of the pump and auxiliary laser, respectively; _γ_ _=_ 1.0 m−1W−1 is the nonlinear coefficient; _θ_ _=_ 0.009 is the coupling rate from

the bus waveguide to the ring cavity; _β__k_ is the _k_-th order dispersion, which utilizes the combinational data from both measurement (the −40th to 40th modes) and FDTD simulations (other

modes), as shown in Fig. 1b. In the model, _α_, _θ_, and _β__k_ are assumed to be identical for the pump and auxiliary lasers. As the comb and auxiliary laser fields counter propagate, XPM

between them is subjected to their average power _P_comb and _P_aux48, imposing a slowly varying nonlinear detuning offset to each other. It is notable that, as can be seen from Equations 1

and 2, when the pump laser enters red-detuning for DKS formation, the XPM induced by the auxiliary laser (2_iγLP_aux) counter balances the increase in the pump detuning _δ_pump and decreases

the self-phase modulation of the pump laser (contained in 2_iγL_|_E_comb|2), which in turn retards the increase in the effective pump detuning. That is, XPM by the auxiliary laser can

expand the pump frequency range where DKS can be accessed, in a similar fashion to that in thermal dynamics (see Supplementary Information Section VIII for more details). Equation (3) above

describes the cavity thermal dynamics, where _α__T_ is the temperature coefficient of the detuning shift (1/0_C_); _C__P_ is the thermal capacity (_J_/0_C_); _K_ is the thermal conductivity

(_J_∙_s_−1∙0_C_−1) of the microcavity to the atmosphere; _Q_ and _Q_abs are the loaded and intrinsic quality factors. respectively36. By fitting the experimentally measured pump

transmissions, we have (_α__T__Q_)_/_(_Q__abs__C__P_) = 5.0e-6 _J_−1 and _K/C__P_ _=_ 7×10−4 _s_−1. We note that the parameters _Q/Q__abs_ and _K_ are set to be larger than the conventional

values in order to simulate the DKS formation process within a reasonable time15. To study the comb dynamics, Eqs. (1–3) is resolved via a split-step Fourier method with a step size of _T_R

(the detailed influences of the thermal effect and auxiliary laser are presented in Supplementary Information Section VII). To acquire the existence range and effective detuning for DKS

within the parameter space of pump power and detuning _δ_pump, we adopt a singlet soliton seeding method using the LLE model. This is achieved by starting the simulation with a hyperbolic

secant pulse as the initial intracavity waveform, whose width and peak power are approximately configured according to the analytical solution1,14. First, we let the initial pulse

numerically evolve under a fixed pump detuning for 10,000 roundtrip times (≈328× of the cavity photon lifetime) to find out the smallest detuning _δ_0,m that allows the initial pulse to

become a DKS without incurring parametric oscillation. This value for _δ_0,m is the lower limit of the DKS existence range for the corresponding pump power level. Afterwards, a pump scanning

simulation is conducted for the initial pulse, by using _δ_0,m as the starting value and linearly increasing it at a rate of 3e-6/_T_R until the DKS pulse vanishes. Δ_P_comb/Δ_δ_ is

recorded for each incident pump power, from which the DKS effective detuning condition is clearly revealed by the sign of the derivative curves, as shown in Fig. 2b. REFERENCES * Akhmediev,

N. General theory of solitons. in Soliton-driven Photonics (eds Boardman, A. D. & Sukhorukov, A. P.) (Dordrecht, Springer, 2001), 371–395. * Herr, T. et al. Temporal solitons in optical

microresonators. _Nat. Photon._ 8, 145–152 (2014). Article  ADS  Google Scholar  * Kippenberg, T. J. et al. Dissipative Kerr solitons in optical microresonators. _Science_ 361, eaan8083

(2018). Article  MathSciNet  Google Scholar  * Huang, S. W. et al. Mode-locked ultrashort pulse generation from on-chip normal dispersion microresonators. _Phys. Rev. Lett._ 114, 053901

(2015). Article  ADS  Google Scholar  * Brasch, V. et al. Photonic chip–based optical frequency comb using soliton Cherenkov radiation. _Science_ 351, 357–360 (2016). Article  ADS 

MathSciNet  Google Scholar  * Yi, X. et al. Soliton frequency comb at microwave rates in a high-_Q_ silica microresonator. _Optica_ 2, 1078–1085 (2015). Article  Google Scholar  * Yao, B. C.

et al. Gate-tunable frequency combs in graphene–nitride microresonators. _Nature_ 558, 410–414 (2018). Article  ADS  Google Scholar  * Yu, M. J. et al. Mode-locked mid-infrared frequency

combs in a silicon microresonator. _Optica_ 3, 854–860 (2016). Article  Google Scholar  * Pfeiffer, M. H. P. et al. Octave-spanning dissipative Kerr soliton frequency combs in Si3N4

microresonators. _Optica_ 4, 684–691 (2017). Article  Google Scholar  * Lee, S. H. et al. Towards visible soliton microcomb generation. _Nat. Commun._ 8, 1295 (2017). Article  ADS  Google

Scholar  * Huang, S. W. et al. A broadband chip-scale optical frequency synthesizer at 2.7 × 10−16 relative uncertainty. _Sci. Adv._ 2, e1501489 (2016). Article  ADS  Google Scholar  *

Huang, S. W. et al. Smooth and flat phase-locked Kerr frequency comb generation by higher order mode suppression. _Sci. Rep._ 6, 26255 (2016). Article  ADS  Google Scholar  * Yang., Q. F. et

al. Stokes solitons in optical microcavities. _Nat. Phys._ 13, 53–57 (2017). Article  Google Scholar  * Herr, T. et al. Mode spectrum and temporal soliton formation in optical

microresonators. _Phys. Rev. Lett._ 113, 123901 (2014). Article  ADS  Google Scholar  * Guo, H. et al. Universal dynamics and deterministic switching of dissipative Kerr solitons in optical

microresonators. _Nat. Phys._ 13, 94–102 (2017). Article  Google Scholar  * Obrzud, E., Lecomte, S. & Herr, T. Temporal solitons in microresonators driven by optical pulses. _Nat.

Photon._ 11, 600–607 (2017). Article  Google Scholar  * Karpov, M. et al. Raman self-frequency shift of dissipative Kerr solitons in an optical microresonator. _Phys. Rev. Lett._ 116, 103902

(2016). Article  ADS  Google Scholar  * Yi, X. et al. Single-mode dispersive waves and soliton microcomb dynamics. _Nat. Commun._ 8, 14869 (2017). Article  ADS  Google Scholar  * Yang, Q.

F. et al. Counter-propagating solitons in microresonators. _Nat. Photon._ 8, 560–564 (2017). Article  Google Scholar  * Yu, M. J. et al. Breather soliton dynamics in microresonators. _Nat.

Commun._ 8, 14569 (2017). Article  ADS  Google Scholar  * Lucas, E. et al. Breathing dissipative solitons in optical microresonators. _Nat. Commun._ 8, 736 (2017). Article  ADS  Google

Scholar  * Webb, K. E. et al. Experimental observation of coherent cavity soliton frequency combs in silica microspheres. _Opt. Lett._ 41, 4613–4616 (2016). Article  ADS  Google Scholar  *

Wang, P. H. et al. Intracavity characterization of micro-comb generation in the single-soliton regime. _Opt. Express_ 24, 10890–10897 (2016). Article  ADS  Google Scholar  * Bao, C. Y. et

al. Observation of Fermi-Pasta-Ulam recurrence induced by breather solitons in an optical microresonator. _Phys. Rev. Lett._ 117, 163901 (2016). Article  ADS  Google Scholar  * Cole, D. C.

et al. Soliton crystals in Kerr resonators. _Nat. Photon._ 11, 671–676 (2017). Article  ADS  Google Scholar  * Zhou, H. et al. Stability and intrinsic fluctuations of dissipative cavity

solitons in Kerr frequency microcombs. _IEEE Photon. J._ 7, 3200113 (2015). Google Scholar  * Marin-Palomo, P. et al. Microresonator-based solitons for massively parallel coherent optical

communications. _Nature_ 546, 274–279 (2017). Article  ADS  Google Scholar  * Geng, Y. et al. Terabit optical OFDM superchannel transmission via coherent carriers of a hybrid chip-scale

soliton frequency comb. _Opt. Lett._ 43, 2406–2409 (2018). Article  ADS  Google Scholar  * Liang, W. et al. High spectral purity Kerr frequency comb radio frequency photonic oscillator.

_Nat. Commun._ 6, 7957 (2015). Article  Google Scholar  * Brasch, V. et al. Self-referenced photonic chip soliton Kerr frequency comb. _Light.: Sci. Appl._ 6, e16202 (2017). Article  Google

Scholar  * Suh, M. G. & Vahala, K. J. Soliton microcomb range measurement. _Science_ 359, 884–887 (2018). Article  ADS  Google Scholar  * Trocha, P. et al. Ultrafast optical ranging

using microresonator soliton frequency combs. _Science_ 359, 887–891 (2018). Article  ADS  Google Scholar  * Suh, M. G. et al. Microresonator soliton dual-comb spectroscopy. _Science_ 354,

600–603 (2016). Article  ADS  Google Scholar  * Dutt, A. et al. On-chip dual-comb source for spectroscopy. _Sci. Adv._ 4, e1701858 (2018). Article  ADS  Google Scholar  * Yu, M. J. et al.

Silicon-chip-based mid-infrared dual-comb spectroscopy. _Nat. Commun._ 9, 1869 (2018). Article  ADS  Google Scholar  * Carmon, T., Yang, L. & Vahala, K. J. Dynamical thermal behavior and

thermal self-stability of microcavities. _Opt. Express_ 12, 4742–4750 (2004). Article  ADS  Google Scholar  * Yi, X. et al. Active capture and stabilization of temporal solitons in

microresonators. _Opt. Lett._ 41, 2037–2040 (2016). Article  ADS  Google Scholar  * Brasch, V. et al. Bringing short-lived dissipative Kerr soliton states in microresonators into a steady

state. _Opt. Express_ 24, 29312–29320 (2016). Article  ADS  Google Scholar  * Joshi, C. et al. Thermally controlled comb generation and soliton modelocking in microresonators. _Opt. Lett._

41, 2565–2568 (2016). Article  ADS  Google Scholar  * Li, Q. et al. Stably accessing octave-spanning microresonator frequency combs in the soliton regime. _Optica_ 4, 193–203 (2017). Article

  Google Scholar  * Stone, J. R. et al. Thermal and nonlinear dissipative-soliton dynamics in Kerr-microresonator frequency combs. _Phys. Rev. Lett._ 121, 063902 (2018). Article  ADS  Google

Scholar  * Herr, T. et al. Universal formation dynamics and noise of Kerr-frequency combs in microresonators. _Nat. Photon._ 6, 480–487 (2012). Article  ADS  Google Scholar  *

Jaramillo-Villegas, J. A. et al. Deterministic single soliton generation and compression in microring resonators avoiding the chaotic region. _Opt. Express_ 23, 9618–9626 (2015). Article 

ADS  Google Scholar  * Erkintalo, M. & Coen, S. Coherence properties of Kerr frequency combs. _Opt. Lett._ 39, 283–286 (2014). Article  ADS  Google Scholar  * Grudinin, I. et al.

Compensation of thermal nonlinearity effect in optical resonators. _Opt. Express_ 19, 7365–7372 (2011). Article  ADS  Google Scholar  * Jost, J. D. et al. All-optical stabilization of a

soliton frequency comb in a crystalline microresonator. _Opt. Lett._ 40, 4723–4726 (2015). Article  ADS  Google Scholar  * Huang, S. W. et al. Globally stable microresonator Turing pattern

formation for coherent high-power THz radiation on-chip. _Phys. Rev. X_ 7, 041002 (2017). Google Scholar  * Del Bino, L. et al. Symmetry breaking of counter-propagating light in a nonlinear

resonator. _Sci. Rep._ 7, 43142 (2017). Article  ADS  Google Scholar  * Suh, M. G. & Vahala, K. Gigahertz-repetition-rate soliton microcombs. _Optica_ 5, 65–66 (2018). Article  Google

Scholar  * Liao, M. L. et al. Enhanced single cavity soliton generation in Kerr microresonators via inverse-Kelly sideband. _IEEE Photon. J._ 9, 4501709 (2017). Google Scholar  * Bao, C. Y.

et al. Spatial mode-interaction induced single soliton generation in microresonators. _Optica_ 4, 1011–1015 (2017). Article  Google Scholar  * Kelly, S. M. J. Characteristic sideband

instability of periodically amplified average soliton. _Electron. Lett._ 28, 806–807 (1992). Article  Google Scholar  * Savchenkov, A. A. et al. Kerr frequency comb generation in overmoded

resonators. _Opt. Express_ 20, 27290–27298 (2012). Article  ADS  Google Scholar  * Matsko, A. B. et al. Chaotic dynamics of frequency combs generated with continuously pumped nonlinear

microresonators. _Opt. Lett._ 38, 525–527 (2013). Article  ADS  Google Scholar  * Lucas, E. et al. Detuning-dependent properties and dispersion-induced instabilities of temporal dissipative

Kerr solitons in optical microresonators. _Phys. Rev. A._ 95, 043822 (2017). Article  ADS  Google Scholar  * Coen, S. et al. Modeling of octave-spanning Kerr frequency combs using a

generalized mean-field Lugiato-Lefever model. _Opt. Lett._ 38, 37–39 (2013). Article  ADS  Google Scholar  Download references ACKNOWLEDGEMENTS The authors acknowledge helpful discussions

with Tatsuo Itoh, Xingyu Zhou, Baicheng Yao, Abhinav Kumar Vinod, Jinghui Yang, and Hao Liu. The authors acknowledge VLC Photonics S.L. and LiGenTec SA for device fabrication. This work is

supported by the National Key R&D Program of China (2018YFA0307400), NFSC grant 61705033, the 111 project (B14039), Lawrence Livermore National Laboratory contract B622827, the Office of

Naval Research award N00014–16–1–2094, and the National Science Foundation awards 1741707, 1810506 and 1824568. AUTHOR INFORMATION Author notes * These authors equal contribution eqally:

Heng Zhou, Yong Geng AUTHORS AND AFFILIATIONS * Key Lab of Optical Fiber Sensing and Communication Networks, University of Electronic Science and Technology of China, 611731, Chengdu, China

Heng Zhou, Yong Geng, Wenwen Cui & Kun Qiu * Fang Lu Mesoscopic Optics and Quantum Electronics Laboratory, University of California, Los Angeles, CA, 90095, USA Shu-Wei Huang & Chee

Wei Wong * Department of Electrical, Computer, and Energy Engineering, University of Colorado, Boulder, CO, 80309, USA Shu-Wei Huang * Institute of Fundamental and Frontier Sciences,

University of Electronic Science and Technology of China, 611731, Chengdu, China Qiang Zhou Authors * Heng Zhou View author publications You can also search for this author inPubMed Google

Scholar * Yong Geng View author publications You can also search for this author inPubMed Google Scholar * Wenwen Cui View author publications You can also search for this author inPubMed 

Google Scholar * Shu-Wei Huang View author publications You can also search for this author inPubMed Google Scholar * Qiang Zhou View author publications You can also search for this author

inPubMed Google Scholar * Kun Qiu View author publications You can also search for this author inPubMed Google Scholar * Chee Wei Wong View author publications You can also search for this

author inPubMed Google Scholar CORRESPONDING AUTHORS Correspondence to Heng Zhou or Chee Wei Wong. ETHICS DECLARATIONS The authors declare that they have no conflict of interest.

SUPPLEMENTARY INFORMATION SUPPLEMENTARY FILE RIGHTS AND PERMISSIONS OPEN ACCESS This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use,

sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative

Commons license, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated

otherwise in a credit line to the material. If material is not included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds

the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/. Reprints and

permissions ABOUT THIS ARTICLE CITE THIS ARTICLE Zhou, H., Geng, Y., Cui, W. _et al._ Soliton bursts and deterministic dissipative Kerr soliton generation in auxiliary-assisted

microcavities. _Light Sci Appl_ 8, 50 (2019). https://doi.org/10.1038/s41377-019-0161-y Download citation * Received: 24 October 2018 * Revised: 10 May 2019 * Accepted: 14 May 2019 *

Published: 29 May 2019 * DOI: https://doi.org/10.1038/s41377-019-0161-y SHARE THIS ARTICLE Anyone you share the following link with will be able to read this content: Get shareable link

Sorry, a shareable link is not currently available for this article. Copy to clipboard Provided by the Springer Nature SharedIt content-sharing initiative